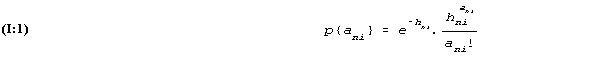
1. In the beginning of the 60'es I introduced a new - or rather a more definite version of an old - epistemological concept. I preserved the name of objectivity for it, but since the meaning of that word has undergone many changes since its Hellenic origin and is still, in everyday speech as well as in scientific discourse, used with many different contents, I added a restricting predicate: specific.
Let it be said at once: my professional background is mathematical and statistical, not philosophical. The concept has therefore not been carved out in a conceptual analysis, but on the contrary its necessity has appeared in my practical activity as a statistical consultant for about 30 years and in the later years as a professor of Theoretical Statistics with Reference to its Applications to the Social Sciences.
During these activities I was introduced to very diverse subjects: medicine and hygiene with the connected parts of biology; psychology and education; technology: economics; demography and sociology; linguistics; etc.
In spite of the diversity of subjects the analytic methods generally available were rather limited for the first many years. But in 1951 I was faced with a task the solution of which added a new tool to my arsenal.
2. In that year The Danish Ministry of Social Affairs wanted an investigation of the development of reading ability in 125, then about 20 years old, former students of public schools in Copenhagen, who in their school years had suffered from serious reading difficulties and therefore had received supplementary education in that discipline.
For each of these students were recorded the results of repeated oral reading tests during his school years - both as regards reading speed and reading accuracy - as well as of a test at an after-examination late in 1951.
It would be a fairly simple task to follow the development of a student's reading ability over a number of years if the same part of the same test were used every time, but at each testing it was necessary to choose a test which corresponded approximately to his standpoint, so as a rule a student was followed up with a series of tests of increasing "degrees of difficulty".
In a concrete formulation of this problem I imagined - in good statistical tradition - the possibility that the reading ability of a student at each stage, and in each of the two above-mentioned dimensions, could be characterized in a quantitative way - not through a more or less arbitrary grading scale, but by a positive real number defined as regularly as the measurement of a length.
Whether this would be possible with the tests in question could not be known in advance. It had to be tried out through a separate experiment which was carried out in January 1952. In this experiment about 500 students in the 3rd-7th school year were tested with 2 or 3 of the texts used in the investigation of students with reading difficulties. With a view to investigate reading accuracy, numbers of reading errors were recorded for each test. As regards reading speed, numbers of words read within a time limit were also recorded.
If we order the persons by their total number of reading errors in two "adjacent" tests, a small segment of the results may be presented in a table of this shape:
| Table 1. Misreading of 10 persons in two tests. | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Person no. | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | Person total |
| Test no. 1 Test no. 2 |
0 0 |
2 2 |
4 2 |
4 9 |
8 7 |
5 14 |
8 20 |
12 17 |
18 15 |
10 26 |
69 115 |
| Test total | 0 | 4 | 6 | 13 | 15 | 19 | 28 | 29 | 33 | 36 | 184 |
As an introductory survey of these data I compared graphically each person's number of reading errors in the two tests for all persons who had had both of them. Figure 1 shows the diagram for the data of Table 1 with a cross for each person (numbered by the letter n), his results in the two tests denoted ani and an2 being plotted as abscissa and ordinate respectively.
Each diagram of this type (with approximately 100 pairs of observations) showed that the numbers of misreadings compared were "by and large" proportionate to each other, although with a wide margin for variations.
3. For these variations we sought to introduce a model of random variability, as we conceived the misreading of a given word by a given student as a haphazard event to which it might be possible to assign a probability - low for easy words, higher for difficult words. [i.e., inverse probability!] And in analogy to probability models for the throwing of dice, it was assumed that whether he actually read a word correctly or not should have no influence on his probability of misreading any other word.
Further, if a test is well chosen, the student should neither have no reading errors nor be totally unable to read the text - that is, the probability of misreading a single word should be fairly low.
In a model of this kind a mathematical deduction leads to expressing the probability of a given number of misreadings for a given student by one of the classical distribution functions, the Poisson distribution.
Stated mathematically, (readers who are not mathematically trained may pass lightly over the formulas here and later on and still be able to follow the argument), the probability that person no. n has ani misreadings in test no. i is
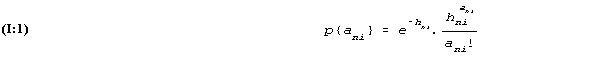
in which appears a "parameter" hni the value of which determines the probabilities of the possible numbers of reading errors, ani.
If the value of the parameter is 0 a result of no errors will occur with a probability of 1 while all other results have probabilities of 0. If the value of the parameter is small, the low numbers of reading errors will have large probabilities and high numbers have small probabilities; if the parameter value is high, the largest probabilities will be found at numbers in some neighborhood of the parameter value.
4. In a given test a good reader will in general make fewer errors than a weak reader, h will be lower; likewise, a student will as a rule have fewer errors in an easy test than in a difficult one.
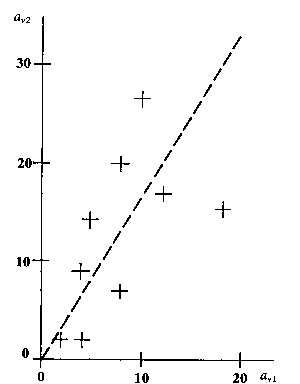
Figure 1. Misreadings in test 2 plotted against misreadings in test 1.
If it would have been possible to draw Figure 1 showing the parameter values, hn1 and hn2 as coordinates instead of the observed numbers of errors, an1 and an2, which are subject to random variations, the points might be expected to fall exactly on a line of proportionality, and thus the ratio between hn1 and hn2 to be the same for all persons; we can choose one person as a reference person and give him the number "0" and obtain
![]()
This formula can be written as
![]()
which should be valid for all pairs of "adjacent" tests and not only for nos. 1 and 2. Thus we get
![]()
and accordingly
![]()
for any of the tests and for any person. From this it appears that the Poisson parameter hni can be written as the product of a person parameter, hn1/h01, which we shall denote as zn, and a test parameter h0i, which we shall denote by Ei. Thus we get [infinite divisibility = conjoint additivity =]
![]()
For a given person (no. n) hni increases with increasing values Ei, meaning larger numbers of reading errors to be expected. It is thus reasonable to speak of this parameter as the "degree of difficulty of the test". Likewise zn can be spoken of as the "degree of disability of the student" or perhaps as his "degree of reading weakness".
1. Obviously it is not a small step from figure 1 to the Poisson distribution (I:1) with the parameter decomposition (I:6). I readily admit that I introduced this model with some mathematical hindsight: I realized that if the model thus defined were proven adequate, the statistical analysis of the experimental data, and thus the assessment of the reading progress of the weak readers, would rest on a solid - and furthermore mathematically rather elegant - foundation.
Fortunately the experimental results turned out to correspond satisfactorily to the model which became known as The Multiplicative Poisson Model [First published by Rao (1952) p. 207, though without any applications, then used as a basic tool in Ellerman et al. (1952). Treated in detail in Rasch (1960).] and which has shown itself applicable in several fields, such as:
Infant mortality in Denmark decreasing sharply over a period of 30 years, as dependent on year and sex;
Suicide frequency in Denmark as related to age, sex and geographic region;
Traffic injuries suffered by individuals as dependent on a person factor (accident-proneness)
and the length of the period of observation;
Traffic accidents on road units as related to road and time period.
2. The outcome of the reading test experiment was beyond expectation: a statistically very satisfactory analysis on the basis of a new model which represented a genuine innovation in statistical techniques!
But the understanding of what the model entails tarried several years. At the 1959 anniversary of the University of Copenhagen the highly esteemed Norwegian economist Ragnar Frisch - later Nobel Prize winner - was to receive an honorary doctorate. I visited him by appointment the next day, and when our business was finished he asked me what I had been doing in the 25 years since I stayed at his institute in Oslo for a couple of months to study a new technique of statistical analysis that he had developed.
As mentioned I had been doing some very varied things, but I soon concentrated on the comparison of reading abilities, one of the topics of a monograph which I was then preparing.
On this occasion I did not mention reading errors, but rather the students' reading speeds. The model is, however, the same: the Multiplicative Poisson Model which I then proceeded to explain to Ragnar Frisch in the following way: [Again readers may pass lightly over the formulas.]
Applied to reading speed the model of (I:1) and (I:6) states that the probability that person no. n in a given time reads ani words of text no. i is determined by the Poisson distribution (I:1) with the parameter value given by (I:6), thus

where in this case a high value of the parameter hni=zn.Ei means that many words are read in the given time, and thus a high value of zn means high reading speed and a large value of Ei means a text which is quickly read, thus in this respect an easy reading test.
Likewise the probability that the person reads anj words of test no. j under similar conditions is
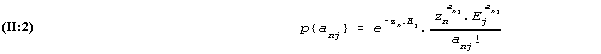
It is also assumed that (the actual results of test no. i, ani, has no influence on the probabilities of the possible outcome of the other test. The multiplication rule for probabilities in these conditions says that the probability of the outcomes ani and anj of the two tests is the product of the two probabilities (II:1) and (II:2) thus
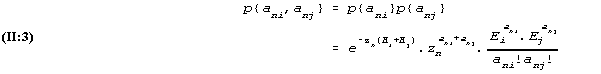
The Poisson distribution has the property (which in fact can be derived from (II:3)) that the sum of the two Poisson distributed variables is also Poisson distributed with a parameter which is the sum of the two parameter values. With the notation an+ for the sum ani+anj, we then have
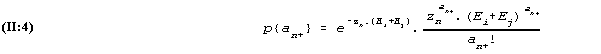
At this stage we apply another basic rule of the calculus of probabilities which in this context can be formulated thus: In the class of possible outcomes where the total number of words read, an+, has a fixed value, the probability of the outcomes ani and anj, conditional on this total, is given by dividing (II:4) into (II:3).
3. Until now the non-mathematical reader has been advised to skip the formulas (and until this point Frisch had only listened politely), but now I shall present a crucial point which demands a careful inspection of the two last formulas, but not necessarily an understanding of their content.
On the right side of (II:3) and (II:4) the person parameter zn appears in exactly the same way in the two expressions, namely in the exponential function of the same argument:
![]()
and raised to the same power:
![]()
When (II:4) is divided into (II:3) these two factors cancel, and the resulting conditional probability does not contain the person parameter zn. The probability that the given number of words read, an+, is composed of ani and anj words of the two tests is expressed by
![]()
which is determined by the observed numbers ani and anj and by the ratio between the difficulty parameters of the two tests, while it is not influenced at all by which person is involved.
In less mathematical language this can be illustrated as follows:
If we group together the persons who have read exactly 100 words of the two tests together, and if the tests
are exactly equally difficult, Ei=Ej, thus Ei/(Ei+Ej)=.5, the chance that for a given person the 100 words
consist of 55 words of test no. 1 and 45 of test no. 2 is the same as the chance of obtaining exactly 55 heads and
45 tails in 100 throws of a "fair coin". The value of this probability is given by
![]()
as can be looked up in standard statistical tables.
If test no. j is twice as difficult as test no. i, so that Ei/(Ei+Ej)=2/3, the probability of the same outcome is
![]()
In practice, of course, the ratio between the two degrees of easiness is not known, but it follows from (II:7) that it can be estimated preliminarily from the two observed numbers, thus
![]()
Such an estimate, based on the results of only one person, is of course very unreliable, but the formula also tells that an estimate can be formed by summing the observed results from several persons.
However, these details are of a technical statistical nature and not essential to our discussion of principles.
On seeing (II:7) Frisch opened his eyes widely and exclaimed: "It (the person parameter) was eliminated, that is most interesting!" And this be repeated several times during our further conversation. To which I of course agreed every time - while I continued reporting the main results of the investigation and some of my other work.
Only some days later I all of a sudden realized what in my exposition had caused this reaction from Ragnar Frisch. And immediately I saw the importance of finding an answer to the following question: "Which class of probability models has the property in common with the Multiplicative Poisson Model, that one set of parameters can be eliminated by means of conditional probabilities while attention is concentrated on the other set, and vice versa?"
1. What Frisch's astonishment had done was to point out to me that the possibility of separating two sets of parameters must be a fundamental property of a very important class of models. I introduced in my first lectures for students of social science (see Lecture Notes, ed. by U. Christiansen, 1963 & 1966,vol. 3) the term `Models for measuring' for this class. [A better name for it might be "Additive Exponential Models". Just as (II:1) is an elaboration of the Poisson distribution, known since 1837, the Models for Measuring are an elaboration in the same direction of the class of distributions called the Darmois-Koopmans exponential family, and discussed extensively since 1935, cf. Barndorff-Nielsen (1973) and Lehman (1959)]. And in publications from 1960 and 1961 I tried to relate this separability to some more general requirements which constitute a first step in carving out the concept of specific objectivity, launched in the lectures. [See Rasch (1960),. Ch. 7 and Rasch (1961), Para. 5.]
In the experiment on reading ability an individualization of psychological testing was achieved by the results of the same student in two or more tests being compared.
In other experiments each person was presented with a certain series of questions to each of which his response was characterized as either right or wrong, or by some other dichotomy. The models used for these responses was that the probabilities of the two possible responses (+ and -) were
![]()
where zn is the "ability parameter" for person n, and Ei the "easiness" parameter for question no. i.
2. Both models are treated in "Probabilistic Models for Some Intelligence and Attainment Tests" (Copenhagen, Danish Institute for Educational Research, 1960; Chicago, MESA Press, 1992), but only a later article "An individualistic approach to item analysis" in P. F. Lazarsfeld and N. W. Henry, (eds.): Readings in Mathematical Social Science. Science Research Associates, Chicago, (1966), discusses the background for the elementary model (III:1) to greater depth, introducing and motivating the concept of specific objectivity which in fact is closely related to R. A. Fisher's concept of "sufficiency":
"As regards the basic formulas (for the model II:) we have already noted that when they are applied to the total set of data they enable us to separate the estimation of one set of parameters from the other. However, (one basic formula) can also be applied to any subgroup of the total collection of subjects having been exposed to the stimuli. Thus the parameters of the subjects in the subgroup can be evaluated without regard to the parameters of the other subjects.
In particular the parameters of any two subjects can be compared on their own virtues, quite irrespective of the group or population to which for some reason they may be referred. Thus the new approach, when applicable, does rule out populations from the comparison of individuals.
Similarly, (another basic formula) can be applied to any subset of the stimuli, and accordingly their parameters can be evaluated without regard to the parameters of the other stimuli. In particular, the parameters of any two stimuli can be compared separately.
With these two additional consequences for the techniques of estimation and model control, the principle of separability leads to a singular objectivity in statements about both parameters and model structure. In fact, the comparison of any two subjects can be carried out in such a way that no other parameters are involved than those of the two subjects, neither the parameter of any other subject nor any of the stimulus parameters. Similarly, any two stimuli can be compared independently of all other parameters than those of the two stimuli, the parameters of all other stimuli as well as the parameters of the subjects having been replaced with observable numbers.
It is suggested that comparisons carried out under such circumstances be designated as "specifically objective". The same term would seem appropriate for statements about the model structure that are independent of all parameters specified by the model, their unknown values being, in fact, irrelevant for the structure of the model."
In connection with a presentation of the general class of models with separability of parameters, the concept is discussed in a later publication (Rasch, 1968, pp. 29-30), where some consequences of the absence of specific objectivity are pointed out:
"Firstly the failing of specific objectivity means that the conclusion about, say, any set of person parameters will depend on which other persons are also compared. As a parody we might think of the comparison of the volumes of a glass and a bottle as being influenced by the heights of some of the books on a shelf.
Secondly, the conclusions about the persons would depend on just which items were chosen for the comparison, a situation to which a parallel would be that the measurement of the relative height of two persons would depend on whether the measuring stick was calibrated in inches or in centimeters.
Avoiding such irrelevant dependencies is just my reason for recommending the use of the models for measuring whenever they may be utilized."
But the need for stating the concept more precisely became urgent, and a first attempt was made in Rasch (1966c) from where I quote two passages:
"2. Humanities and Natural Sciences.
When first suggesting the models (for measuring) I could offer no better excuse for them than their apparent suitability, which showed in their rather striking mathematical properties. In Rasch (1961) a more general point of view was indicated, according to which the models were strongly connected with what seemed to be basic demands for a much needed generalization of the concept of measurement.
In continuation of that paper my attention was drawn to other fields of knowledge, such as economics, sociology, history, linguistics, evaluations of arts, where claims are arising of being taken just as seriously as Natural Sciences.
On a first sight the observational material in Humanities would seem very different from that in physics, chemistry and biology, not to speak of mathematics. But it might turn out that the difference is less essential than it would seem. In fact, the question is not whether the observations are of very different types, but whether Sciences could be firmly established on the basis of quite different types of observation.
3. Scientific Statements: Comparisons, Being Objective.
Such considerations led to the question from which I now start my inquiry: What is Science? Which conditions must be fulfilled so that a statement can be qualified as scientific, thus competing with those in Natural Sciences?
That science should require observations to be measurable quantities is a mistake, of course: even in physics observations may be qualitative (e.g., emission of radio-active particles recorded as number of scintillations on a susceptible screen) as in last analysis they always are! (e.g., as reading off a point as located between two marks on a measuring rod).
Two features seem indispensable in scientific statements:
1) They deal with comparisons
2) The comparisons must be objective.However, to complete these requirements I have to specify the kind of comparisons and the precise meaning of objectivity. When doing so, I do not feel confident that all sorts of what justifiably can be called "science" are covered, but certainly a very large area is."
1. During a discussion in a seminar on objectivity problems it was pointed out that my theory of specific objectivity was strongly tied to the concept of comparisons.
I admitted that this was the case, but I have to add that to my mind comparisons form an essential part of our recognition of our surroundings: we are ceaselessly faced with different possibilities for action, among which we have to choose just one, a choice that requires that we compare them. This holds both in everyday life and in scientific studies.
Firstly consider a somewhat artificial example from "daily life". I have in front of me two ashtrays. One is rather heavy, not bad in appearance, while the other is lighter and more elegant, but decisive for me is a third property, whether they are solid enough for the handling they may easily get exposed to in my parlor.
If I want reliable information on this matter I must test them by a suitable sequence of exposures, e.g., letting them fall to the floor from different heights, e.g., two rather low, H1 and H2, two rather high, H5 and H6, and two middle, H3 and H4. Each time it is observed whether the ashtray is damaged or not (- or +). [To avoid a cumulative effect it may be better to use a new specimen from the production line of each ashtray for each test, disregarding "for the sake of argument" any inaccuracy caused by variations in the products.]
The results may be arranged in the following way:
| Falling distance | |||||||
| H1 | H2 | H3 | H4 | H5 | H6 | ||
| (IV:1) | Heavy ashtray | + | + | + | + | - | - |
| Light ashtray | + | + | - | - | - | - | |
The low and high falling distances will be seen not to reveal any difference between the ashtrays, while the middle distances do show the heavy ashtray to be more solid than the light one.
This result I may make use of when buying a new ashtray.
The example is artificial and primitive, but still gives a couple of methodological hints:
1.1. To determine whether an object has a certain property one must do something specific to the object, confront it with some action or different actions liable to create one of a number of reactions.
1.2. If knowledge gained in this way is to be used in making a choice it must be obtained for several objects of the kind in question so that a comparison becomes possible.
2. That comparisons are also ubiquitous in scientific discourse was mentioned in III in connection with the question "What is Science?" where it was pointed out that scientific statements deal with comparisons, and they were required to be "objective".
Let us elucidate this by discussing a law of elementary physics, the general gas equation, assumed valid under idealized, but not unrealistic conditions. It says that when a given amount of an ideal gas at a certain moment has volume v under pressure p at an "absolute temperature" of T, then these three quantitative properties must obey the balance equation
![]()
where the constant r characterizes the given amount of the given gas.
Obviously, this law deals with comparisons in the sense that it enables them: it is possible to determine what happens to pressure when either temperature is changed and volume kept constant, or vice versa.
The results from such variations can be entered into a diagram similar to (IV:1)
| V1 | V2 | . | . | . | . | ||
| T1 | p11 | p12 | . | . | . | . | |
| (IV:3) | T2 | p21 | p22 | . | . | . | . |
| . | . | . | . | . | . | . | |
| . | . | . | . | . | . | . |
where according to the law (IV:2):
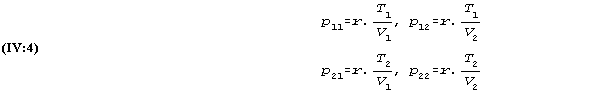
A comparison of the two temperatures T1 and T2 with respect to their effects on pressure for constant volume shows that
![]()
which is the same for all volumes.
In the same way one can compare the volumes v1 and v2 with respect to their effect on pressure for constant temperature:
![]()
which is the same for all temperatures.
3. In modern physics the law (IV:2) can be derived from a general theory of temperature and pressure as resulting from the random movements of an immense number of molecules in a closed space, but it was actually found purely empirically without referring to such a theory. Let us examine how that might have come about.
Firstly, it seems obvious that the pressure of a given amount of a gas on the enclosing walls must be influenced both by changes in volume and in temperature. By varying these two factors systematically one may perform an experiment like the one described in (IV:2) recording corresponding values of pressure (p), volume (v) and temperature (t), the last measured in one of the standard scales, e.g., Centigrade, since we do not assume an advanced concept as "absolute temperature" (T) known at this stage. The results are set up in a diagram like (IV:3).
If we first keep the volume constant and plot the pressures p11, p21, ... found in the first column of the diagram against the corresponding temperatures t1, t2, ..., the picture strikes one as a linear correspondence, say
![]()
This relationship is conceived as valid for all temperatures in the interval determined by the lowest and the highest temperature included in the experiment, thus in a way as a general law.
But on the other hand: if it is only valid for one particular volume it is very special and thus of little use.
We therefore choose another constant volume p2 and again find a linear relationship, but with different constants:
![]()
On closer inspection it may strike one that the ratio a2 / b2 is very nearly the same as a1 / b1. However, this as well as the linearity itself could be incidental, but it may encourage us to try a more extensive series of constant volumes v2,...vn.
This shows the linearity to be a pervading trait and the a's plotted against b's to give near proportionality. Thus one may assume a/b to be nearly a constant (in the following denoted by k). In this connection we may consider that measurement errors do occur, and besides the law itself may not be completely exact.
But b is certainly not a constant, it decreases markedly with increasing volume, and closer inspection reveals it to be inversely proportionate to the volume - practically speaking - the constant of b.v is denoted r.
Thus we have found a law of greater generality than (IV:7a and b), namely
![]()
which, within some interval, holds for all volumes and temperatures.
This is, however, also of some particularity, since it only holds for a given amount m of the gas (m standing for the mass of the amount).
This situation calls for repetitions of the experiment with different amounts of gases m1, m2, ... to see whether (IV:8) holds every time. It actually does, and k even has the same value each time!
But r varies with m, and a new graphical analysis reveals the relationship to be a proportionality, so we shall write
![]()
We have now obtained a very general gas equation:
![]()
but it is peculiar to the particular gas examined.
To abolish this limitation we must investigate several gases and for each ascertain that (IV:10) still holds, and then study what happens to the "constants" q and k.
The first result is that k is really a constant, the same for all gases, about -273 Centigrade, and we therefore introduce
![]()
as the "absolute temperature". It is customarily measured in the "Kelvin Scale", that with the same unit as the Centigrade scale, but with a zero point of - 273.16 Centigrade which in a larger theoretical framework is identified as the point where every matter loses its identity.
On the other hand q is peculiar to each composite gas, but may still be characterized in a general way. To do this, consider that a pure gas consists of an immense number of similar molecules with the same weight M. It turns out that q is inversely proportionate to M, thus
![]()
where the factor R is a universal constant, the "Gas constant".
On inserting (IV:11) and (IV:12) into (IV:10) we then, for a pure gas, get
![]()
which is usually written
![]()
Here the factor
![]()
expresses the amount of the pure gas as a multiple of the molecular weight; when that is measured in grams the unit of n is the so-called grammolecule or mole.
However, for a composite gas the grammolecules of the components simply add up to the total amount of gas measured in these units.
Since also the pressure of the components are additive - while volume and temperature are common to all of them - it follows that (IV:14) also holds for composite gases.
And so we have got a really general gas equation, holding for any gas if the limits of the ideal state is not approached. [This discussion is based upon Holton & Roller (1958), Chs. 21 and 25.]
4. The detailed analysis described above started from various prescientific observations of volume, pressure and temperature - undoubtedly made already in antiquity - and passed through a series of stepwise comparisons:
1) of different temperatures with respect to their influence on the pressure of a given amount of a certain gas contained in a given volume;
2) of different volumes for each temperature, given a constant amount of the gas;
3) of different amounts of the same gas for each combination of temperature and volume;
4) of different pure and composite gases for each combination of amount, volume and temperature.
In each step a general law valid within a certain area of variability was searched for, that is, the observational result (pressure) was compared for different values of 1) temperature, 2) volume, 3) mass and 4) composition, in order to ascertain a general rule, such as a proportionality.
In each of the first three steps the rule is of increasing, but still limited generality, and in the fourth step we reach the general law valid for all gases under "ideal conditions", that is in a state where the molecules, as it were, are not "too close".
And within each step the structure and scope of the rule were established through a series of internal comparisons, while the transition from one step to the next entailed an extension of the scope and a concomitant external comparison.
This procedure, it seems, can be taken as a prototype of the experimental charting of a complex field. [For a substantial simplification of it, see VII.]
But the principle of charting through comparisons is not limited to the experimental sciences. In fact. without being able to experiment with the celestial bodies, Kepler could compare the apparent orbits of the planets and find structures which covered at least the period of Tycho Brahe's observations and which proved valid for all the known planets. And this was one of the starting points for Newton's derivation of the fundamental laws for the movements of all sorts of bodies.
This decisive phase in the development of classical mechanics thus, in the last resort, depended on comparisons. And in the next centuries the scope of these laws was delimited through further comparisons.
It is my opinion that only through systematic comparisons - experimental or observational - is it possible to formulate empirical laws of sufficient generality to be - speaking frankly - of real value, whether for furthering theoretical knowledge or for practical purposes.
On this basis I see systematic comparisons as a central tool in our investigation of the outer world. Accordingly I shall, in the following, attempt a closer analysis of the concept of comparison.
1. In order to generalize and formalize the preceding arguments I shall now make the presentation more abstract.
Let us imagine two collections of elements, O and A, denoted here objects and agents. The collections may contain a finite number of elements or be infinite. Single elements are denoted O and A, respectively, and may carry indices, e.g. On, or Ai, without regard to whether the collections are enumerable or not.
Every object O of O may enter into a well-defined contact C with every agent A of A, and every such contact has an "outcome" R. From a formal viewpoint the contact C is nothing but the pair of elements (O,A). The collection of possible contacts is C, and the collection of possible different outcomes is denoted R.
In some cases there are only two possible outcomes, like + and - in the ashtray experiment, or Heads and Tails in coin throwing. In other cases there is a larger finite number, such as in questionnaires with forced choices between, e.g., 4 possible answers. In still other cases all positive real numbers may be the outcomes, often de facto limited to a closed interval, as in the case of measuring heights of persons. Many other types of collections of outcomes are possible.
The specification of the three collections of elements gives the frame of reference,
![]()
within which we shall now define the concept of comparison. [To facilitate later extensions, objects and agents are also denoted as factors, and the frame of reference F, (V:1) is therefore spoken of as bifactorial.]
2. In a frame of reference where each contact C determines the outcome R uniquely, this outcome is called determinate, and is denoted a reaction. It may then be considered as a function of the object O and the agent A which form the contact, and may be written
![]()
To avoid misunderstandings I shall point out that objects and agents O and A are often qualitative concepts, and the same may be the case for R. The reaction function is thus not a mathematical function (as, e.g., a power function or an exponential function), but only a single-valued correspondence such that to every element C=(O,A) in the collection of contacts corresponds one and only one element R in the collection R of possible reactions.
Under this condition the frame of reference is denoted as determinate, a qualification that should he understood in the strict mathematical sense as explained, and not to be confounded with philosophical concepts related to the term "determinism".
3. Within a specified determinate frame of reference, a comparison between two objects O1 and O2 - with regard to their reactions to containing the agent A - is defined as a statement about them which is based solely on those reactions
![]()
to contacting A.
A statement is in the first instance defined as the "value" of a function of R1 and R2 which is defined for all pairs of elements in R. The "values" of this comparing function u(R1,R2) form a collection U, the elements of which may - as those of R - be qualitative.
If we insert for the arguments of the function u the expressions in (V:3) it is seen that the value
![]()
is really a statement about O1 and O2. But it is obvious that what it says will, in general, depend on A, the agent used in the comparison. We can therefore view the comparing function u(R1,R2) as a function of O1 and O2, conditioned by A: accordingly we write
![]()
The function v we denote as a comparator for O1 and O2 conditioned by the agent A (in analogy to the concept of conditional probability).
With an arbitrarily chosen comparing function we may easily get different answers for different A's. Thus in the ashtray example the conclusion for falling distance H1, H2, H5, H6 would be that the ashtrays are equally solid, but for intermediate distances like H3 or H4 that the heavy ashtray is more solid. Both conclusions are formally correct, each statement is dependent on the agent; in such cases we shall speak of local comparisons.
Local comparisons may very well be useful as pointers, but they are local and do not function in comparisons within the total frame of reference in question.
4. For a comparing statement (V:5) about O1 and O2 to be more than locally valid, the comparator must be independent of which A from A has been used to produce the reaction.
If this condition is fulfilled we denote the comparison between these two objects as global for agents which are members of A and then the function (V.5) can be written more simply as
![]()
5. However, if this globality within A holds for any two objects O1 and O2 in O, we shall characterize pairwise comparisons of objects as defined by (V:4) as specifically objective within the frame of reference F.
The term "objectivity" refers to the fact that the result of any comparison of two objects within O is independent of the choice of the agent A within A and also of the other elements in the collection of objects O; in other words: independent of everything else within the frame of reference than the two objects which are to be compared and their observed reactions.
And the qualification "specific" is added because the objectivity of these comparisons is restricted to the frame of reference F defined in (V:1). This is therefore denoted as the frame of reference for the specifically objective comparisons in question.
This also makes clear that the specific objectivity is not an absolute concept, it is related to the specified frame of reference.
It also deserves mention that this definition concerns only comparisons of objects, but within the same frame of reference it can be applied to comparisons of agents as well. In cases where specific objectivity holds for comparison of the objects, it does not necessarily hold for comparisons of the agents, although the two often go together.
In the ashtray example the objects are ashtray types, numbering 2 elements, the agents are falls from different distances, numbering 6, and the reactions are + (holds) and - (breaks), numbering 2. A possible comparing function could be the assertion "No. 1 is more solid than no. 2", defined operationally by the sequence of reactions + -, that is, the first one holds and the second one breaks. The comparison is not global, it has the value "true" for the intermediate falling distances and "false" for the others.
Another comparing function is "no. 1 is at least as solid as no. 2", defined operationally by the observed reactions ++ or +-: either they both hold or they both break or only no 1. holds. This comparison is global within the frame of reference of the described experiment and can even be expected to be global also if more ashtray types and more falling distances are included in the frame of reference.
VI. Realization of specific objectivity in bifactorial frames of reference.
1. From the preceding argument it appears that specific objectivity is not to be expected from an arbitrarily chosen comparing function u(R1, R2).
On the contrary, for a given frame of reference F, one must ask if there at all exists such a function. A general answer to this question cannot yet be given, but a class of bifactorial frames of reference can be indicated for which the answer to the question is rather simple. This class, very common in physics, but also known on other quantitative sciences, has the property that every object and every agent as well as every reaction is characterized by a so-called scalar parameter, that is a real number characteristic of the object, agent or reaction.
The parameters for On, Ai and Rni may be denoted bn, di and xni, respectively.
Since the reaction is assumed to be uniquely determined by object and agent, xni is a single-valued function of bn and di
![]()
This function we shall call the parametric reaction function.
The condition corresponding to (V:6) for specific objectivity of comparison of objects, that is of bm and bn, is
![]()
In analyzing this equation we shall assume the functions u and q - and thus also v - to have convenient mathematical properties. [Continuity of the said functions and of their first order derivatives with respect to each of their respective arguments.]
2. Under these conditions a decisive statement can be made on the properties of the reaction function q(b,d) that are necessary for establishing specific objectivity of comparisons of objects within the framework F.
Likewise it is possible to indicate the necessary structure of the comparator v(bm,bn).
These statements must, however, be preceded by a remark on the freedom inherent in the choice of parameters.
If for instance b is positive for all objects of O an O may as well be characterized by
![]()
as by b because there is a one-to-one relationship between b and b' in contrast to the situation where b could assume both negative and positive values, in which case to one b' would correspond both to b and -b.
The example illustrates the general principle that b may be replaced by any monotonic function b' of b:
![]()
since the one-to-one relationship holds for such functions.
The same of course also holds for d and x.
By way of such transformations it is possible to substitute one parameter system by another which may he more convenient.
3. We can now formulate one of the main theorems of the theory of specific objectivity:
Let objects and agents in the bifactorial determinate frame of reference F be characterizable by scalar parameters b and d, and reactions by a scalar reaction function of "convenient" mathematical properties
![]()
Then the existence of three (strictly) monotonic functions
![]()
transforming the parametric reaction function (VI:5) into a purely additive relation
![]()
will be necessary and sufficient condition for specifically objective comparability of objects as well as agents.
If such functions exist, they are unique apart from trivial linear transformations.
The specifically objective comparison of two objects Om and On and of two agents Ai and Aj is based on the respective differences
![]()
and
![]()
the right-hand expressions of which are denoted: the elementary comparators.
If this condition is satisfied, the frame of reference is called latently additive, while the comparisons between objects or between agents are called latently subtractive.
In passing, one may observe that comparisons by means of the elementary comparators constitute interval measurements on the b'- and d'-scales. A closer analysis of the relationship, thus hinted at, between the two concepts: measurement and comparison seems called for.
4. As a step in the treatment of the general gas equation in IV,3, we analyzed an imagined experiment in which the amount of a given gas was kept constant, while the pressure p (measured, e.g., in dynes per cm2) is seen as the result of the interaction between the volume v (cm3) as the object and the temperature t (Centigrade) as the agent, while both the composition of the gas and its mass are kept constant.
All three variables are real numbers, p and r are necessarily positive, while t may be negative.
The experiment leads to the relationship (IV:8), which, written as
![]()
can be taken as the reaction function in a frame of reference denoted as
![]()
with v (belongs to V), t (belongs to T) and p (belongs to P) as scalar parameters.
Main theorem I says that for specifically objective comparisons of either volumes or temperatures - with respect to their effect on pressure - to be possible, the reaction function (VI:10) must be latently additive.
As it stands (VI:10) is multiplicative, but logarithmizing makes it additive
![]()
Later experiments have shown -k to be a universal constant, the absolute zero of temperature.
The transforming functions are thus
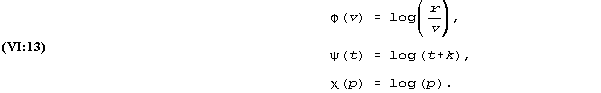
The comparisons between two volumes and between two temperatures are described in IV,2. where proof is given of their independence of temperature and volume respectively, and thus of specific objectivity within the frame of reference (VI:11).
As demonstrated by Borchsenius (1977) similar situations are encountered everywhere in classical physics, and the generality inherent in this is essential for the broad applicability of physics.
1. Main theorem I deals with reactions produced through contacts between two kinds of elements, denoted objects and agents, corresponding to the methodological view-points expressed in IV,I, that to obtain knowledge of whether an object has a certain property - or to which degree it has it - one must expose it to a series of agents which reveal the property.
Systematic investigations are often structured as such interactions between two factors with all other factors of possible influence kept constant. That was the case in the first of the experiments on ideal gases described in IV,3. which led to (IV:8).
But even if we succeed - as in this case - in finding the necessary latent additivity, the possibility will still exist that the relationship obtained changes its character totally with a new value of one of the factors kept constant.
It is therefore necessary to extend our concepts to frames of reference where the reactions are produced by an interaction between more than two types of elements. [i.e, the many-facet Rasch model]
2. For the sake of simplicity we shall at first limit ourselves to determinate reactions to interaction between three factors or agents. [To the terminology "object" and "agent" we shall return in VIII in connection with processes.]
For the possible reactions and the collection of them we keep the designations R and R, while the three collections of agents are denoted A, B, and C with elements A, B and C. The set of the four collections is the frame or reference
![]()
An R is conceived as a single-valued reaction function r of the three agents
![]()
A comparison of agents A1 and A2 for given B and C is defined as a single-valued function of the two corresponding reactions
![]()
thus
![]()
as the comparing function and v as the comparator for A1 and A2, conditional on B and C (cf. (V:5)).
This defines a local comparison, and if the comparing function u has been chosen in such a way that the result (VII:4) of the comparison is the same for all Choices of B and C we have a global comparison of A1 and A2.
If moreover a global comparison exists for all pairs of elements of A such comparisons are specifically objective within the frame of reference given by (VII:1), and we may write
![]()
[The more complex concept of a comparison of two pairs of agents (A1, B1) and (A2, B2), local or global with respect to different C's, which could also be introduced, we shall leave out of this exposition.]
3. As for bifactorial frames of reference it will often, in concrete instances, be reasonable to characterize both the agents A, B, C and the reaction R by scalar parameters which we denote as a, b, c, and x.
The reaction parameter x is then assumed to be a function of the agent parameters:
![]()
will, "convenient" mathematical properties. It is denoted the parametric reaction function.
Parameterizing in a corresponding way to the requirements of (VII:2) for specifically objective comparisons within F of any two A-parameters a1 and a2 with the parametric reaction functions
![]()
requires the existence of a "convenient" comparing function
![]()
which for all combinations of parameter values representing elements of F is independent of b and c, thus depending only on a1 and a2:
![]()
4. A main theorem analogous to I can be derived for the existence of a comparing function u leading to specifically objective comparisons for each of the three dimensions:
If the agents of a trifactorial frame of reference (VII:1) can be characterized by scalar parameters a, b and c, and if the reaction function (VII:6) has convenient mathematical properties then the existence of four (strictly) monotonic functions
![]()
transforming (VII:6) into an additive relation
![]()
will be a necessary and sufficient condition for specific objectivity of comparisons within each of the collections A, B and C.
[This important theorem is due - in a much more general form - to Borchsenius (1974).]
An obvious corollary to Main Theorem I is that specific objectivity of the objects entails specific objectivity of agents, and vice versa. The corresponding corollary to Main Theorem II is that specific objectivity of comparisons within two of the three collections of agents entails specific objectivity within the third.
5. In the final shape (IV:13) of the general gas equation the pressure
![]()
depends on three factors: volume, temperature, and amount of gas in question.
The condition stated in Main theorem II for specific objectivity of comparisons of the values of each of the three scalar parameters n, T and v - with respect to their effect on pressure - are fulfilled as we see by the logarithm of (VII:12):
![]()
which equation is additive in the transformed parameters
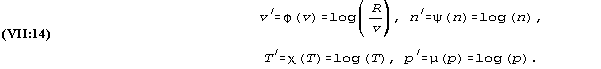
6. In IV an outline was drawn of an experiment whose first step was to record the pressure corresponding to different volumes and temperatures of a given amount of a given gas. The next step was to study how this relationship was changed with variations of the amount of the gas. Eventually it became necessary to experiment with various pure gases as well as with mixtures of different gases; in the last instance both of the last variations were combined into one parameter: the number n expressing the amount of gas in terms of grammolecules.
Taken literally this procedure would lead to a tremendous amount of complex experiments. But in practice one may neglect this and only study a few factors at a time, form a picture of the overall situation and test this by a number of well-chosen cases.
The reason that such a piece-wise procedure may lead to the correct result lies in the following two facts:
1) The fundamental laws of physics usually allow specific objectivity of comparisons within each component.
2) The relevant parameters for a given problem are almost always scalar.
In fact Main Theorem II may be supplemented by a third main theorem which we shall also formulate for trifactorial frames of reference only, but the extension to more than three factors is straightforward.
Let a0, b0 and c0 be arbitrarily chosen fixed values of the scalar parameters A, b, and c of a trifactorial frame of reference [a, b, c, x] with a scalar reaction function (VII:6) of convenient mathematical properties. Then all of the three partial bifactorial frames of reference
![]()
are latently additive. If this property also holds for the trifactorial frame of reference, then the transformations needed in the bifactorial frameworks must - but for trivial additive constants - coincide with those required in the trifactorial case.
Thus the problem of transforming to latent additivity in tri- and actually in multi-factorial frames of reference as well, is reduced to the same problem concerning a limited number of bifactorial frames of reference, followed up by comparing "synonymous" transformations and, if they prove satisfactory, by some well chosen extra checks, possibly even covering all available data.
7. In the experimental design for obtaining the complete general gas equation we may thus draw the conclusion, that as regards transformations the simultaneous crossing of all three factors described in IV,3-4 can be replaced by variations of two factors at a time, the third factor being kept constant each time:
1) For fixed amount n of any gas the pressure p is latently additive in temperature t and volume v.
2) For fixed volume v of a given gas the pressure p is latently additive in its amount n and temperature t.
The main theorems II and III then state that if p is latently additive in all three factors then the transformations needed in the two trifactorial frames of reference are the same as those leading to additivity in the bifactorial frames of reference, viz. those presented in (VII:14).
As a safeguard one may also perform the experiment with the third pair of factors and obtain the control of the model inherent in a comparison of the two determinations of the transforming function for each parameter and of the three determinations of the transformations of the reaction p.
8. One question of importance remains: How, after a series of bifactorial experiments, one finds out in which way it is necessary to transform each of the variables, e.g., as in a sub-case of the general gas equation, that all the variables have to be transformed logarithmically - though in the case of temperature not until some particular constant (k) has been added to the value as measured in one of the conventional scales (Centigrade, Reamur or Fahrenheit).
The practical solution of this problem usually turns out to be rather easy by means of a theorem that has been presented (in Danish) in Rasch (1972), but as this is mainly a technical question we shall not enter into it here.
1. The present paper is only meant as an introduction to the idea of specific objectivity - IV-V to the basic concepts and VI-VII to its immediate implications.
The exposition in these sections has therefore been limited to static frames of reference with determinate outcomes of interactions, firstly between two types of factors (objects and agents), later between more interacting factors. In VI and VII both factors and reactions are assumed amenable to scalar parameterizations, but it may be remarked that there exists some parallels to Main Theorems I-III for the case where the parameters are sets of numbers, "vectors", if they all have the same number of elements (dimension).
The concept of specific objectivity,however, has applications beyond these limitations.
In this section we shall indicate how the concept of specific objectivity can be introduced into a dynamic system by way of a bifactorial frame or reference
![]()
2. By a (determinate) static system of reference we shall mean a frame of reference (VIII:1) in which the reaction to one contact does not influence the reaction to any other contact. As, e.g., in V,2 where the reaction R2 of O2 to A is not influenced by how O1 reacted to A - or by how a third O reacted to another A. So far this has been implicit in the formulation of the procedure of comparing.
In this chapter we shall touch upon the problem of specific objectivity in dynamic systems where the reaction to a given contact may influence other contacts, exemplifying it, however, only by situations in which each object contacts the elements of A in a fixed order and where each reaction may influence only the next contact directly. But it may be mentioned that more general cases are readily tractable.
3. As an introductory example let me describe the main features of an experiment with pig feeding [Source: Ludvigsen & Thorbek (1959)].
14 pigs were bought at birth and the experiment started when they were taken from their mothers at an age of 20 days. In the experimental period, lasting till their age of 64 days , they were fed on the same synthetic food material, but with different supplements to each of three experimental groups K-I, K-II, and K-III.
Each day all pigs were offered the same ration, but of course everyone ate according to need, and digestion and combustion were also personal processes within the chemical and physiological laws governing them.
The growth of each pig was followed by weighings about every fifth day of the experimental period as shown in Table 2 for 3x2 of them, none of which were siblings.
Analyses of several such experiments have shown that after transforming the weight Vit (i: pig no., t: age minus 20 days) to their logarithms:
![]()
it is possible to determine an age parameter gt, called the growth mode, common for all pigs, such that vit plotted against gt shows almost perfect linearity for each pig, which is then fully characterized by two individual parameters: the location ai and the slope bi. The latter is called the individual growth rate:
![]()
| Table 2. Growth of 6 piglets from three different experimental series. | ||||||
|---|---|---|---|---|---|---|
| Age | Live weights: Vit (kg.) | |||||
| in days | K-I,24 | K-I,60 | K-II,6 | K-II,11 | K-III,24 | K-III,21 |
| 20 | 4.0 | 4.8 | 4.5 | 5.1 | 5.0 | 4.8 |
| 25 | 4.9 | 6.3 | 6.1 | 6.6 | 6.7 | 6.6 |
| 30 | 6.2 | 7.4 | 7.9 | 8.1 | 8.5 | 8.1 |
| 34 | 7.5 | 8.8 | 9.2 | 9.6 | 9.6 | 9.1 |
| 38 | 8.9 | 10.0 | 10.5 | 10.4 | 11.0 | 10.6 |
| 42 | 10.2 | 12.1 | 12.5 | 12.1 | 12.8 | 12.1 |
| 48 | 12.7 | 15.1 | 14.9 | 14.7 | 15.3 | 15.2 |
| 53 | 15.1 | 16.8 | 17.6 | 17.6 | 18.6 | 17.4 |
| 59 | 16.8 | 19.1 | 19.7 | 19.2 | 19.9 | 18.1 |
| 64 | 16.6 | 20.9 | 22.0 | 22.4 | 23.1 | 22.0 |
| Table 3. Logarithmic transform of the observed weights (vit = loge Vit) | |||||||
|---|---|---|---|---|---|---|---|
| Age | Logarithms of live weights: vit = loge Vit | Growth mode | |||||
| in days | K-I,24 | K-I,60 | K-II,6 | K-II,11 | K-III,24 | K-III,21 | vi |
| 20 | 0.60 | 0.68 | 0.65 | 0.71 | 0.70 | 0.68 | 0.670 |
| 25 | 0.69 | 0.80 | 0.79 | 0.82 | 0.83 | 0.82 | 0.792 |
| 30 | 0.79 | 0.87 | 0.90 | 0.91 | 0.93 | 0.91 | 0.885 |
| 34 | 0.88 | 0.94 | 0.96 | 0.98 | 0.98 | 0.96 | 0.950 |
| 38 | 0.94 | 1.00 | 1.02 | 1.02 | 1.04 | 1.03 | 1.008 |
| 42 | 1.01 | 1.08 | 1.10 | 1.08 | 1.11 | 1.08 | 1.077 |
| 48 | 1.10 | 1.18 | 1.17 | 1.17 | 1.18 | 1.18 | 1.163 |
| 53 | 1.18 | 1.23 | 1.25 | 1.25 | 1.27 | 1.24 | 1.237 |
| 59 | 1.23 | 1.28 | 1.29 | 1.28 | 1.30 | 1.26 | 1.273 |
| 64 | 1.22 | 1.32 | 1.34 | 1.35 | 1.36 | 1.34 | 1.322 |
| Increase of vit | 0.62 | 0.64 | 0.69 | 0.64 | 0.66 | 0.66 | 0.652 |
| Growth rate | 0.951 | 0.982 | 1.058 | 0.982 | 1.012 | 1.012 | - |
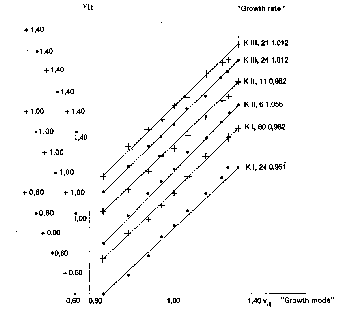
Figure 2.Logarithmic growth of 6 piglets during about 1½ months related
to the average logarithmic growth of the whole batch of 14 piglets.
As shown in fig. 2 the said linearity holds - apart from small deviations that look unsystematic - in the whole experimental period for each of the 6 pigs.
For an orientation we may then consider growth as a perfectly determinate process, thus neglecting the said variations, taking the straight lines connecting the first and the last point for each piglet as representing its exact growth process.
Accordingly the parameters of (VIII:3) are identified with empirical values such as (after normalization):
![]()
where "." stands for averaging over the 14 experimental pigs.
However unambiguous this result is, it does not permit specifically objective comparisons between the parameters for two pigs by means of their weights at the same time; as a matter of fact there exists no comparing function of
![]()
which is independent of the age parameter gt. - Nor do the weights of the same pig at two ages allow a comparison of the two age parameters which is independent of the parameters of the pig.
However, the proper subject of the study is not the single weights of the animals, it is their growth, that is: the progress of weights. From a biological point of view it is obvious that growth from age t1 to age t2 has something to do with the size of the pig at the first age: accretion of weight at a given time t occurs by way of production and destruction of cells, both on the basis of the existing cells. A tentative assumption of proportionality for this relationship will lead to an attempt at expressing growth as a relative increase, that is by means of the ratio
![]()
In fact, an increase in vt as defined by (VII:2) can be expressed as
![]()
thus being a transformation of the relative increase.
The question of possible specific objectivity of such comparisons may, as in the case of growth of pigs, be answered through modifications of the theory of V-VII and corresponding modifications of the main theorems.
The same holds for generalizations to much more complex processes, with interactions of several factors, with states that are influenced not only by the immediate preceding state, but by several or all previous states.
Main Theorem I states that specifically objective comparability of pigs as well as of age levels in a scalar parameterization with respect to growth requires the reaction parameter, i.e., vt+1-vt, to be latently additive in terms of one parameter for the pig and one for the age level.That this is actually the case follows from (VIII:3) since
![]()
where dt=gt+1-gt. And (VIII:8) is multiplicative, thus latently additive.
4. The growth process defined by (VIII:8) is a special case of a class of determinate dynamic systems where every object Oi of a collection goes through a series of states in which they are confronted with a sequence of agents A1, ..., Ai, ... .
It is assumed that each object is characterized by a permanent parameter bn and initially in a state On0 with a parameter value xn0. Under the influence of the agent A1 with the parameter value a1 the state is changed from On0 to On1 with the parameter xn1 determined by xn0 and a1, but also by the permanent parameter bn, in a relationship which may be written
![]()
This function is called the transition function.
After that the object, now in state On1, is confronted with the agent A2 with parameter a2, which changes xn1 into
![]()
where xn1 is given by (VIII:9). And so on.
This referential system gives occasion for attempting two sorts of comparisons:
Between the permanent parameters of different objects on the basis of their state parameters at the same two or more stages of the process.
Between two agent parameters on the basis of two or more state parameters for the same object.
Comparisons between the initial states of two objects on the basis of later states of the objects do not give rise to specific objectivity.
1. In IV-VIII the exposition has concentrated on determinate frames of reference. In the analysis of empirical data this at best means an idealization of the situation, which in the case of both the general gas equation and the growth of pigs gave rather precise descriptions of the actual data. But of course the physical example contains at least measurement errors and the biological case also small capricious variations in the digestive processes of pigs.
In physics, however, there also occur "genuine" uncontrollable responses, e.g., in radioactive radiation where the number of emissions per time unit - counted for instance by means of luminous points on a sensitive screen - varies just as "randomly" as the reading errors of school children in a text. After Planck's discovery of the quantum of energy and Niels Bohr's understanding of the hydrogen spectrum as produced by random shifts of the orbits of electrons, the whole development of atomic theory has been governed by the concept of random events - not totally lawless, but ruled by probabilities inherent in the models.
The mapping of all indeterminacy of responses on the concept of probability - which actually is derived from "mere toys" like games of roulette, dice and cards - is today not only prevalent in large areas of physics, but also in biology and in social science.
For the concept of specific objectivity to be of use it is therefore essential that it can be applied to indeterminate - more concretely: to probabilistic - frames of reference.
2. A treatment of this topic corresponding to the treatment in IV-VIII of determinate frames of reference must await a later occasion. But the case which awoke Ragnar Frisch's intense curiosity may illustrate the problem.
As mentioned in I, in comparing the numbers of misreadings of each student in two reading tests I found very large and apparently capricious variations. But it was possible to map this indeterminacy on the Poisson probability distribution (I:1), and its parameter was in I,4 shown to be decomposable into a product of a factor for the student and a factor for the test, expressed in the formula (II:1).
By somewhat involved algebraic deductions I obtained from this formula the fundamental result (II:7) which states that for the two indeterminate responses of a student to two tests, viz., his numbers of misreadings, an1 and an2, we may derive a probabilistic statement which involves the two test parameters E1 and E2, but is independent of the student parameter zn. This statement may therefore be characterized as specifically objective: if does not depend on anything unknown in the frame of reference except the two parameters that are to be compared.
3. Let us invert the problem.
Starting from the Poisson distribution (I:1) with the parameter hni, characteristic of the contact between n and i, this parameter is a determinate reaction to the contact. Moreover it is scalar: if we further assume that persons and tests can be characterized by scalar parameters which we denote as zn and Ei, respectively, it follows directly from Main Theorem I for determinate frames of reference that the reaction parameter hni must be latently additive - or equivalently, latently multiplicative - in these parameters. Thus hni may be taken as some function of the product zn.Ei, say
![]()
Then it can be shown that the function h must be the identity function, i.e.,
![]()
and thus the rather audacious interpretation in (I:6) of the empirical results outlined in I,2 is replaced by a logical consequence of the epistemological requirement that a testing of the relative reading abilities of students (with regard to reading errorS) should be objective in the sense of being independent of irrelevant circumstances within the given frame of reference.
This argument shows that the Multiplicative Poisson Model is a necessary condition for specific objectivity of comparisons between tests and between students.
But it is also sufficient:
As regards the comparison of tests it follows directly from (II:7) as I explained to Ragnar Frisch - and for the comparison of students (nos. m and n) the analogous result holds:
![]()
which is a probabilistic statement about the reading abilities of the two students that depends only on their observed numbers of misreadings ami and ani on one hand, and on the ratio between their reading abilities on the other.
4. The result that for the Poisson distribution the multiplicative structure (IX:2) of its parameter hni is both necessary and sufficient for obtaining specific objectivity can be extended to the whole class of additive exponential models, touched upon in III,1. In all these models specific objectivity is achieved by means of conditional probabilities, while it is not attainable by marginal probabilities. [Another class of models, formally providing specific objectivity through marginal probabilities, does exist, but has not yet been properly explored in this respect.]
With such an apparatus at one's disposal as a supplement to what was outlined in VII and VIII for determinate frames of reference an arsenal of methods is now available for performing analyses, the result of which are objective in a precise sense. The effectiveness of these methods has been demonstrated in elementary physics by Borchsenius (1977) and tested on empirical data from various fields such as
Psychological test theory
Development psychology
Social psychology
Biology of growth
Economic growth problems
Traffic security research.
Further tests and applications indicated by such tests, as well as further development of the theory and computational techniques, are of course greatly desirable.
The limitation, chosen here, to scalar parameterization will be removed in forthcoming publications, cf. Borchsenius (1974). When extended to multifactorial frameworks the method produces a large class of models for which direct parallels to the three main theorems for determinate static models can be formulated.
Finally these models may also act as building stones in probabilistic dynamic models from which it is possible to draw specifically objective inferences.
Georg Rasch
University of Copenhagen, Denmark
The author wishes to express his gratitude for financial support during many years of research on the topic here presented. Since 1961 funds have been granted by the Carlsberg Foundation, the Rask-Ørsted Foundation and the Danish State Research Foundation, and since 1968 by the Danish Social Science Research Council, which has furthermore financed the translation of the present article from Danish by Mag. art. G. Leunbach and supported its publication.
Published in the Danish Yearbook of Philosophy, Vol. 14, 1977. p. 58-93.
Proceedings of the Symposium in Scientific Objectivity held at "Rolighed", Vedbæk, May
14-16, 1976. Copenhagen: Munksgaard.
Corrected for typographical and translational flaws: MESA Memorandum No. 18
References
Barndorff-Nielsen, O. (1973), Exponential Families and Conditioning, Aarhus Universitet.
Borchsenius, K. (1974), A group theoretical formalization of the concept of objectivity, [Unpublished].
Borchsenius, K. (1977), On specific objectivity and its role in elementary physics. [Unpublished].
Ellermann, M., C. A. Larsen & G. Rasch (1952), Report to the Danish Department Of Social Welfare. (Unpublished).
Holton, G. & D. H. D. Roller (1958), Foundations of Modern Physical Science, Addison-Wesley, Reading, Mass. In particular, Ch. 21: Gases and the structure of matter, pp. 365-376, and Ch. 25: The kinetic theory of matter and heat, pp. 429-460.
Lehman, E. L. (1959), Testing Statistical Hypotheses, Wiley, New York.
Ludvigsen, J. & G. Thorbek (1959), Studies on Growth and Nutrition of Piglets I., Report no. 311 from The Experimental Laboratory, Copenhagen.
Rao, C. R. (1952), Advanced Methods in Biometrics, Wiley, New York.
Rasch, G. (1960), Probabilistic Models for Some Intelligence and Attainment Tests, Danish Institute for Educational Research, Copenhagen; (1992) Chicago: MESA Press.
Rasch, G. (1961), On general laws and the meaning of measurement in psychology, Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, Vol. 4, pp. 321-333.
Rasch, G. (1966a), Lecture Notes, ed. U. Christiansen. (Mimeographed, Copenhagen 1963).
Rasch, G. (1966b), An individualistic approach to item analysis, In: Lazarsfeld. P. F. & N. W. Henry (eds.), Readings in Mathematical Social Science, Science Research Associates, Chicago.
Rasch, G. (1966c), An informal report on the present state of a theory of objectivity in comparisons, Proceedings of the NUFFIC International Summer Session in Science at "Het Onde Hof", The Hague, 14.-28. July.
Rasch, G. (1968), A Mathematical Theory of Objectivity and Its Consequences for Model Construction, Paper read at European Meeting on Statistics, Econometrics and Management Science, Amsterdam 2.-7. September.
Rasch, G. (1972), Objektivitet i samfundsvidenskaberne. Et metodeproblem (Objectivity in Social Sciences. A Methodological Problem), Nationalokønomisk Tidsskrift, pp.161-196.
Postscript
This list covers only the works pertaining most directly to the
content of the present paper. A list representative of the rich
developments in US, Austria, Germany, Australia and Scandinavia is
going to appear in a forthcoming monograph [never published],
planned to give the mathematical theory behind this paper as well
as a variety of applications.
Go to Top of Page
Go to Institute for Objective Measurement Page
| FORUM | Rasch Measurement Forum to discuss any Rasch-related topic |
| Coming Rasch-related Events | |
|---|---|
| Apr. 21 - 22, 2025, Mon.-Tue. | International Objective Measurement Workshop (IOMW) - Boulder, CO, www.iomw.net |
| Jan. 17 - Feb. 21, 2025, Fri.-Fri. | On-line workshop: Rasch Measurement - Core Topics (E. Smith, Winsteps), www.statistics.com |
| Feb. - June, 2025 | On-line course: Introduction to Classical Test and Rasch Measurement Theories (D. Andrich, I. Marais, RUMM2030), University of Western Australia |
| Feb. - June, 2025 | On-line course: Advanced Course in Rasch Measurement Theory (D. Andrich, I. Marais, RUMM2030), University of Western Australia |
| May 16 - June 20, 2025, Fri.-Fri. | On-line workshop: Rasch Measurement - Core Topics (E. Smith, Winsteps), www.statistics.com |
| June 20 - July 18, 2025, Fri.-Fri. | On-line workshop: Rasch Measurement - Further Topics (E. Smith, Facets), www.statistics.com |
| July 21 - 23, 2025, Mon.-Wed. | Pacific Rim Objective Measurement Symposium (PROMS) 2025, www.proms2025.com |
| Oct. 3 - Nov. 7, 2025, Fri.-Fri. | On-line workshop: Rasch Measurement - Core Topics (E. Smith, Winsteps), www.statistics.com |
Our current URL is www.rasch.org
The URL of this page is www.rasch.org/memo18.htm