The Partial Credit Model and the One-Item Rating Scale Model

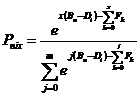
At least one aspect of Rasch measurement continues to perplex analysts and paper reviewers. Are Masters' Partial Credit Model and Andrich's Rating Scale Model variants of the same polytomous model or different models?

The Andrich (1978) Rating Scale Model conceptualizes all items on an instrument to share the same m+1 ordered-category rating scale:

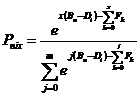
with the usual parameterization, where x=0,m and F₀=0 or any convenient constant, also = 0.

The Masters' (1982) Partial Credit Model conceptualizes each item to exhibit a unique rating scale structure of m_i+1 ordered categories.:

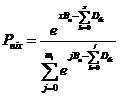
where x=0,m_i and F_i0=0 or any convenient constant.

In many survey instruments, subsets of items share rating scales, some items have unique rating scales, and some items are dichotomies. The generalization of the Andrich Rating Scale Model to groups of items encompasses all these:

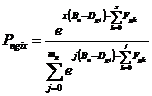
where g indicates the group of items (sharing the same rating scale structure) to which item i belongs.

But what if every group contains only one item? Then g has the same meaning as i, and this model becomes:

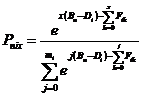
Now it appears that there are two different Rasch models for the identical situation: the "Partial Credit" and the "One-Item Rating Scale". What is the relationship between them? Let us take the Partial Credit model and reparameterize D_ik as b_i + τ_ik where

Then,

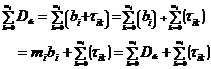
Therefore, which is the same constraint as in the one-item rating scale model.

Thus, the difference between the two models is reduces to parameterization. The "Partial Credit" D_ik is identical to the "one-item Rating Scale" D_i + F_ik as constrained by

and

Consequences of this equivalence include the definition of an overall "item difficulty" for a Partial Credit item as D_i, and also any theoretical properties or practical implications obtained for one model can be carried directly over to the other.

D_i has a convenient interpretation: it is the location (i.e., person measure) on the latent variable at which the highest and lowest category are equally probable. To confirm this, let B_n be the ability of person n with equal probability of being observed in the lowest and highest categories of item i of difficulty D_i:

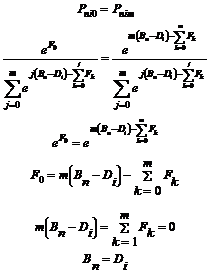
Thus item difficulty for Andrich's Rating Scale model and Masters' Partial Credit model can have the same definition. The models are equivalent.

John Michael Linacre

Andrich D. (1978) A rating scale formulation for ordered response categories. Psychometrika, 43, 561-573.

Masters G.N. (1982) A Rasch model for partial credit scoring. Psychometrika, 47, 149-174.

The Partial Credit Model and the One-Item Rating Scale Model, Linacre J.M. … Rasch Measurement Transactions, 2005, 19:1 p. 1000-1002

Rasch Publications
Rasch Measurement Transactions (free, online)	Rasch Measurement research papers (free, online)	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Applying the Rasch Model 3rd. Ed., Bond & Fox	Best Test Design, Wright & Stone
Rating Scale Analysis, Wright & Masters	Introduction to Rasch Measurement, E. Smith & R. Smith	Introduction to Many-Facet Rasch Measurement, Thomas Eckes	Invariant Measurement: Using Rasch Models in the Social, Behavioral, and Health Sciences, George Engelhard, Jr.	Statistical Analyses for Language Testers, Rita Green
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Journal of Applied Measurement	Rasch models for measurement, David Andrich	Constructing Measures, Mark Wilson	Rasch Analysis in the Human Sciences, Boone, Stave, Yale
in Spanish:	Análisis de Rasch para todos, Agustín Tristán		Mediciones, Posicionamientos y Diagnósticos Competitivos, Juan Ramón Oreja Rodríguez

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt191e.htm

Website: www.rasch.org/rmt/contents.htm