Comparing Item Calibration Estimates using Winsteps and RUMM2010

Stable estimates of item calibration are important and the results should be consistent using different software programs for research to progress satisfactorily. Items tend to greater consistency although produced by person responses (Fisher, 2010). The database of 3,121 persons and 26 items for the KCT-R (Stone, 2002) produced a test separation value of 38.22 corresponding to a test reliability of 0.99. The person separation value was 2.54 corresponding to a person reliability of 0.87. A sample of 260 persons ages 5 - 60 produced a test-retest coefficient of 0.96.

Two programs for producing estimations - Winsteps (Linacre, 2002) and RUMM (Andrich, D., et al., 2000) - were utilized. A well-defined variable and wide item/ability logit range made the KCT-R data useful for this comparison. Newer releases provide additional enhancements, but the basic estimation algorithms remain constant.

Figure 1 is a plot of item calibration estimations using Winsteps and RUMM2010. This figure accents the differences. The Winsteps estimates were lower than the estimates using RUMM2010 for items 1 - 12, and slightly higher for items 13 - 26. A difference of about 0.8 logits was observed for the two easiest items of the test. These differences decreased to item 12. They reversed thereafter with the difference continuing to about 0.3 for the most difficult items.

Figure 2 identifies the stability in the estimations. The lowest four calibration estimates show the largest deviations that diminish towards 0.0 and then increase with the highest two calibration estimates showing the greatest difference. In spite of the noted differences between the two software programs, the item calibration estimates are highly correlated. The wide-range of the calibration estimates contributes to a high r2 of 0.99, but with Winsteps estimates slightly more extreme than RUMM2010 estimates. Stability exists between the two estimations processes with the differences well within any meaningful difference. Nevertheless, a slight difference exists in the estimation process.

Futoshi Yumoto
American Institutes for Research
Collaborative for Research on Outcomes and Metrics
and
Mark Stone
Aurora University, Aurora, IL

Andrich, D., Lyne, A., Sheridan, B., & Luo, G. (2000). RUMM 2010: Rasch Unidimensional Measurement Models. Australia: Rumm Laboratory Try Ltd.

Fisher, W. P., Jr. (2010, 30 September). Distinguishing between consistency and error in reliability coefficients: Improving the estimation and interpretation of information on measurement precision. Social Science Research Network. Retrieved from http://ssrn.com/abstract=1685556.

Linacre, J. M. (2003). Winsteps. Beaverton Oregon: Winsteps.com.

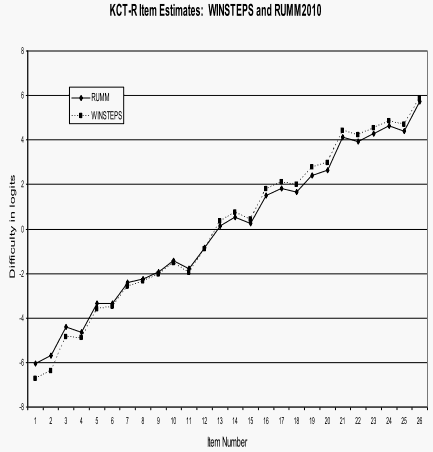
Figure 1. A plot of item calibration estimates using Winsteps and RUMM2010. Points on the solid line are RUMM estimates. Points on the dashed line are Winsteps estimates. The y-axis is "Difficulty in Logits"

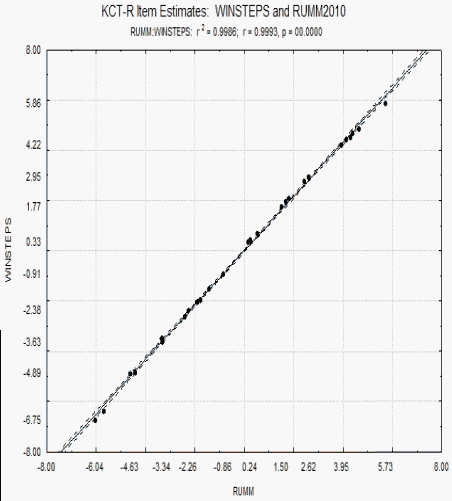
Figure 2. A cross-plot of the KCT-R items calibration estimates using Winsteps (y-axis) and RUMM2010 (x-axis) .

Comparing Item Calibration Estimates using Winsteps and RUMM2010, Futoshi Yumoto & Mark Stone ... Rasch Measurement Transactions, 2011, 25:3, 1329

Rasch-Related Resources: Rasch Measurement YouTube Channel
Rasch Measurement Transactions & Rasch Measurement research papers - free	An Introduction to the Rasch Model with Examples in R (eRm, etc.), Debelak, Strobl, Zeigenfuse	Rasch Measurement Theory Analysis in R, Wind, Hua	Applying the Rasch Model in Social Sciences Using R, Lamprianou	El modelo métrico de Rasch: Fundamentación, implementación e interpretación de la medida en ciencias sociales (Spanish Edition), Manuel González-Montesinos M.
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Rasch Models for Measurement, David Andrich	Constructing Measures, Mark Wilson	Best Test Design - free, Wright & Stone Rating Scale Analysis - free, Wright & Masters
Virtual Standard Setting: Setting Cut Scores, Charalambos Kollias	Diseño de Mejores Pruebas - free, Spanish Best Test Design	A Course in Rasch Measurement Theory, Andrich, Marais	Rasch Models in Health, Christensen, Kreiner, Mesba	Multivariate and Mixture Distribution Rasch Models, von Davier, Carstensen
Rasch Books and Publications: Winsteps and Facets
Applying the Rasch Model (Winsteps, Facets) 4th Ed., Bond, Yan, Heene	Advances in Rasch Analyses in the Human Sciences (Winsteps, Facets) 1st Ed., Boone, Staver	Advances in Applications of Rasch Measurement in Science Education, X. Liu & W. J. Boone	Rasch Analysis in the Human Sciences (Winsteps) Boone, Staver, Yale	Appliquer le modèle de Rasch: Défis et pistes de solution (Winsteps) E. Dionne, S. Béland
Introduction to Many-Facet Rasch Measurement (Facets), Thomas Eckes	Rasch Models for Solving Measurement Problems (Facets), George Engelhard, Jr. & Jue Wang	Statistical Analyses for Language Testers (Facets), Rita Green	Invariant Measurement with Raters and Rating Scales: Rasch Models for Rater-Mediated Assessments (Facets), George Engelhard, Jr. & Stefanie Wind	Aplicação do Modelo de Rasch (Português), de Bond, Trevor G., Fox, Christine M
Exploring Rating Scale Functioning for Survey Research (R, Facets), Stefanie Wind	Rasch Measurement: Applications, Khine	Winsteps Tutorials - free Facets Tutorials - free	Many-Facet Rasch Measurement (Facets) - free, J.M. Linacre	Fairness, Justice and Language Assessment (Winsteps, Facets), McNamara, Knoch, Fan

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt253e.htm

Website: www.rasch.org/rmt/contents.htm