On Temperature

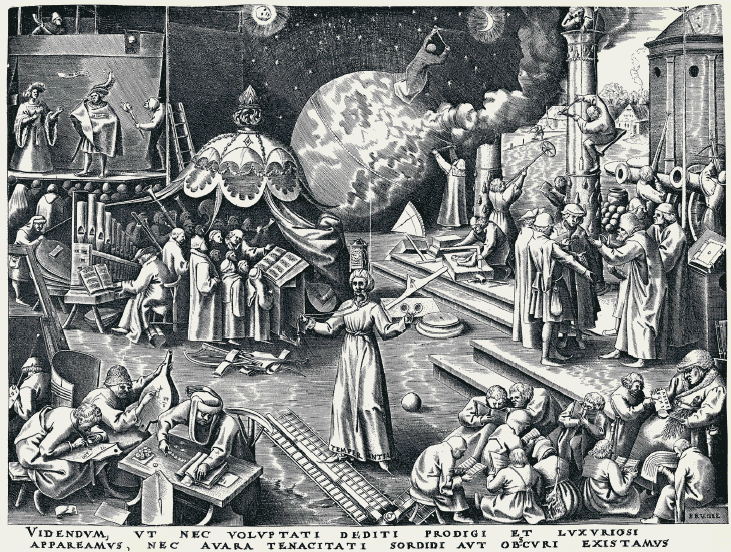
Figure 1: Bruegel's Temperance. From: employees.oneonta.edu/farberas/

The 1560 print of Temperance (Figure above) by Pieter Bruegel, the Elder, illustrates "pantometry" (geometrical measurement) in action. The upper right section of this print portrays practical applications of the mathematical sciences and measurement. These scenes illustrate quantification attempts across many aspects of measuring; using a divider, square, plumb line, visual sighting, aspects of velocity/distance with cannons or crossbow together with disputation also serving a prominent role. (For more about this picture, see Crosby, 1997.)

Quantification and visualization go hand in hand with observations by providing the key to understanding measurement. Arithmetic, geometry, and trigonometry share with writing and music the pursuit of uniform quanta. Writing and music are linear events no less embodying measurement than any other area of science. Bruegel captured more than just the historical scene, he pictures the essence of metrology - a continuous search for units with generality.

Application and usefulness of units requires that all measures (and units) possess sensus communis or "common sense" as Kant (1917) expressed it. Kant meant that communication among peoples is not possible without a "common sense" operating. Visualizing measurement is applying common sense by the use of pictures, graphs, maps, etc. This approach is the key to success in communication, utility and generality (Stone, Wright & Stenner, 1999).

Measurement is always made by means of an analogy. Hans Vahinger (1924) wrote,

All cognition is the apperception of one thing through another ... we are always dealing with an analogy and we cannot imagine how otherwise existence can be understood ... all knowledge can only be analogical. (p. 29)

Common examples from the past for measuring time include the tolling of bells, sundials and water-clocks. Today we have digital watches and atomic clocks for measuring time with greater accuracy. "Time passes," we say. "Time marches on," and when it does we record the duration in terms of length. There is no "time," only duration. Length is the analogy for duration. A theory of time as duration is transformed by analogy from a variable of length and made manifest using natural occurrences such as the sun, moon and stars, and artificial devices as mentioned earlier.

Robert Oppenheimer (1955) in his address to the American Psychological Association entitled Analogy in Science said:

Whether or not we talk of discovery or of invention, analogy is inevitable in human thought, because we come to new things in science with what equipment we have, which is how we have learned to think, and above all how we have learned to think about the relatedness of things. We cannot, coming into something new, deal with it except on the basis of the familiar and the old fashioned. ... We cannot learn to be surprised or astonished at something unless we have a view of how it ought to be; and that view is almost certainly an analogy. (p. 129-130)

Rasch (1961) addressed this problem with a theory, a class of models and specific data examples. His goal was "replacing qualitative observations by quantitative parameters" (p. 331).

Consider temperature and its common measurement. Temperature for most of us means the heat or cold we experience in our environment. In laboratories it is more rigorously studied, but in day-to-day life as well as in scientific laboratories, temperature requires some analogous method by which to make measures. A thermometer commonly uses an expansion tube of mercury to accomplish this task. Water, alcohol among other elements were investigated in arriving at the choice for mercury. Variations abound on the way to utility.

Figure 2. Celsius and Fahrenheit Temperature. From: www.scimathmn.org

Consider the common indoor/outdoor device, the thermometer often showing both Celsius and Fahrenheit: shown in Figure 2.

For practical purposes the thermometer is simply an "expansion tube" of mercury. The elevation (length) of mercury in the tube is analogous to temperature. This elevation is made utilitarian whereby we associate numerals to our personal sensations of comfort/discomfort. Thirty degrees F is experienced as cold and 70 degrees F is considered warm. In countries using Celsius, 0 C and 20 C convey approximately the same sensations. The two scales, C and F, illustrated in the figure are not different. The distance between the two horizontal lines indicating high (red line) and low (blue line) show an equal vertical distance of length on the F and C scales. Any other lines drawn horizontally across the two tubes will indicate exactly the same elevation on both scales.

One intriguing aspect of this instrument is that volume in three dimensions for the thermometer has been reduced to length in one dimension for interpreting temperature. A complex variable has been reduced to a simple one. Rasch (1980) in discussing models in classical physics remarked,

None the less it should not be overlooked that the laws do not at all give an accurate picture of nature. They are simplified descriptions of a very complicated reality" (p. 10, our emphasis).

This point seems rarely appreciated to judge from the voluminous amount of commentary in the social sciences citing how "complicated" reality is, and how difficult it is to model. Physics has progressed admirably following "simple" laws to model complex matters. Scientists appreciate complexity, but nature cannot be understood when complexity is made a stumbling block to understanding. In such instances, emphasizing complexity obfuscates understanding and knowledge. This temperature example reminds us that complexity can be modeled in a simple fashion if only we can find a useful way to do so.

What is different between these two temperature scales is their division of length into segments, each one with different units and different origins for locating zero degrees. Celsius and Fahrenheit report different temperature numerals, but not different temperatures. It is the numerals that differ, not the temperature because the values of C and F can be connected by an algebraic expression, e.g. 9C = 5F - 160. Entering C and solving for F, or vice versa, give us the corresponding value.

A horizontal line across the picture of the temperature tubes supplies all the visual analogy we need to move from one scale to the other. This is because the "height" i.e. the length of mercury in the tube is invariant. It is the same height for each scale. The C and F scales are shown to be equal by observing this line connecting the two lengths. Algebra connects these two different scales precisely. What is not the same are the respective scale divisions and there are numerous variants.

The implications of this simple example can be important for understanding the essence of measuring:

1. We measure by analogy. We have moving hands, clocks ticking, and sand trickling through an hour-glass. No matter how sophisticated the device (cesium clock) analogy prevails in some form. For temperature: The internal liquid of a glass thermometer is a visual representation on the quantitative scale(s).

2. We should not be confused by differing scale values and origins into thinking complexity abounds. A validly constructed instrument emanates from a single, unified variable. The problem is to devise and construct one. For temperature: There is only one construct variable, but many ways to divide and express it.

3. Validity rests on achieving instrument integrity and invariance. Everything else is peripheral to this problem, and only serves to confuse the matter. Constructing the instrument and applying it to life are two entirely different matters not to be confused. For temperature: The instrument is foundational, applications follow.

4. Portability is necessary. Handled properly the instrument is useful in almost all locations. Extreme conditions in temperature or elevation above/below sea level require modifications and corresponding interpretation. For temperature: General application and utility constitute validity with some unique exceptions.

5. Utility is an important aspect of measuring. The choice between two explanations, complex vs. simple (Occam's razor), favors the simple as the useful one. Utility implies understanding. For temperature: Giving one's attention to observing the temperature, and not to the instrument illustrates the successful achievement of utility.

Mark Stone and Jack Stenner

References:
Crosby, A.W. (1997) The Measure of Reality. Chapter 1: Pantometry Achieved. assets.cambridge.org

Kant, I. (1917). Gesammelte Schriften, Vol. 7. Berlin: Reimer. (Original work published in 1798)

Oppenheimer, R. (1956). Analogy in science. American Psychologist, 127-135.

Rasch, G. (1961). On general laws and the meaning of measurement in psychology. Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, 4, 321-333.

Rasch, G. (1980). Probabilistic models for some intelligence and attainment tests. Chicago: The University of Chicago. (Originally published 1960)

Stone, M., Wright, B. & Stenner, J. (1999). Mapping variables. Journal of Outcome Measurement, 3 (4), 306-320.

Vaihinger, H. (1935). The philosophy of "As If". London: Kegan Paul.

On Temperature, M. Stone, J. Stenner, Rasch Measurement Transactions, 2012, 26:1, 1351-3

Rasch-Related Resources: Rasch Measurement YouTube Channel
Rasch Measurement Transactions & Rasch Measurement research papers - free	An Introduction to the Rasch Model with Examples in R (eRm, etc.), Debelak, Strobl, Zeigenfuse	Rasch Measurement Theory Analysis in R, Wind, Hua	Applying the Rasch Model in Social Sciences Using R, Lamprianou	El modelo métrico de Rasch: Fundamentación, implementación e interpretación de la medida en ciencias sociales (Spanish Edition), Manuel González-Montesinos M.
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Rasch Models for Measurement, David Andrich	Constructing Measures, Mark Wilson	Best Test Design - free, Wright & Stone Rating Scale Analysis - free, Wright & Masters
Virtual Standard Setting: Setting Cut Scores, Charalambos Kollias	Diseño de Mejores Pruebas - free, Spanish Best Test Design	A Course in Rasch Measurement Theory, Andrich, Marais	Rasch Models in Health, Christensen, Kreiner, Mesba	Multivariate and Mixture Distribution Rasch Models, von Davier, Carstensen
Rasch Books and Publications: Winsteps and Facets
Applying the Rasch Model (Winsteps, Facets) 4th Ed., Bond, Yan, Heene	Advances in Rasch Analyses in the Human Sciences (Winsteps, Facets) 1st Ed., Boone, Staver	Advances in Applications of Rasch Measurement in Science Education, X. Liu & W. J. Boone	Rasch Analysis in the Human Sciences (Winsteps) Boone, Staver, Yale	Appliquer le modèle de Rasch: Défis et pistes de solution (Winsteps) E. Dionne, S. Béland
Introduction to Many-Facet Rasch Measurement (Facets), Thomas Eckes	Rasch Models for Solving Measurement Problems (Facets), George Engelhard, Jr. & Jue Wang	Statistical Analyses for Language Testers (Facets), Rita Green	Invariant Measurement with Raters and Rating Scales: Rasch Models for Rater-Mediated Assessments (Facets), George Engelhard, Jr. & Stefanie Wind	Aplicação do Modelo de Rasch (Português), de Bond, Trevor G., Fox, Christine M
Exploring Rating Scale Functioning for Survey Research (R, Facets), Stefanie Wind	Rasch Measurement: Applications, Khine	Winsteps Tutorials - free Facets Tutorials - free	Many-Facet Rasch Measurement (Facets) - free, J.M. Linacre	Fairness, Justice and Language Assessment (Winsteps, Facets), McNamara, Knoch, Fan

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt261a.htm

Website: www.rasch.org/rmt/contents.htm