Discrete-time Survival Analysis: A Rasch-Model Logistic Regression

Singer and Willett (S&W, 1993) use a logistic regression model for discrete-time survival analysis that works because it is a Rasch model. They study the drop-out rate for 3,941 special education teachers hired in Michigan between 1972 to 1978.

Their technique and rationale provide support and insight for Rasch practitioners. S&W Model A, reparameterized, is where
P_ni = the probability that teacher n drops out in year i, -D_i = the baseline hazard level in year i, B = the "fixed-effect" proclivity to drop-out, common to all teachers. Set at B=0.

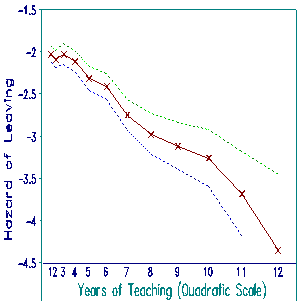Years are encountered sequentially until a teacher drops out or data collection ceases causing the teacher's data to become missing. The S&W teacher survival data for 12 years is depicted in the Figure. The years are rescaled quadratically to clarify the trend. Manual calculation and a Facets Rasch analysis confirm S&W's results.

S&W can take their analysis further by using the measurement properties of their models. Linearity is assured for fitting data because their models incorporate the necessary and sufficient conditions for constructing linear measures. What is not assured is the extent to which their data cooperate in constructing this linearity, i.e., fit their model.

S&W suggest two methods for checking linearity. The first is to partition data and check for parameter invariance. This method is standard in Rasch work. Rasch (1960/1980) uses grouping to look for systematic deviations. His method is widely used to check for differential item functioning.

S&W's second method is to investigate whether better global fit is obtained by adding non-linear predictors. This strategy is disastrous because it sacrifices linearity for better model-to-data fit, degrading the model into a transient description of local data.

Linearity, however, is vital for comprehensibility and generality. Only with linearity does one unit mean the same amount everywhere - only with linearity are graphs readable. Introducing non-linear components produces uneven and hence incomparable units. This disaster is also incurred when a "guessing" parameter is added to a model to "explain" misfitting low ability performances.

Instead of introducing interaction terms to account for misfit, a measurement- oriented strategy examines residual patterns and quality-control fit statistics for useful data partitions. Local failures of the data to support linear measurement construction are detected by local misfits, rather than by the increase in global fit that occurs when local misfits are modelled non-linearly. Identification of local misfit motivates the analyst either to edit the data toward coherent subsets or to reconceptualize the linear model. In the S&W data where "very high-scoring teachers [on the National Teacher's Examination] are disproportionately likely to leave," it makes sense to stratify teachers by NTE score group, just as Rasch (1980 p.96-100) stratified test takers by their speed. Then, since the hazard measures for each score group are in a linear frame of reference, these score group measures can be compared by standard graphical and statistical techniques. Benjamin D. Wright

Singer J. D. & Willett J. B. (1993) It's about time: Using discrete-time survival analysis to study duration and the timing of events. Journal of Educational Statistics. Summer. 18(2) p.155-195.

Discrete-time survival analysis. Wright BD. Rasch Measurement Transactions 1993 7:3 p.307

Discrete-time survival analysis: a Rasch-model logistic regression. Wright BD. … Rasch Measurement Transactions, 1993, 7:3 p.307

Rasch Publications
Rasch Measurement Transactions (free, online)	Rasch Measurement research papers (free, online)	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Applying the Rasch Model 3rd. Ed., Bond & Fox	Best Test Design, Wright & Stone
Rating Scale Analysis, Wright & Masters	Introduction to Rasch Measurement, E. Smith & R. Smith	Introduction to Many-Facet Rasch Measurement, Thomas Eckes	Invariant Measurement: Using Rasch Models in the Social, Behavioral, and Health Sciences, George Engelhard, Jr.	Statistical Analyses for Language Testers, Rita Green
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Journal of Applied Measurement	Rasch models for measurement, David Andrich	Constructing Measures, Mark Wilson	Rasch Analysis in the Human Sciences, Boone, Stave, Yale
in Spanish:	Análisis de Rasch para todos, Agustín Tristán		Mediciones, Posicionamientos y Diagnósticos Competitivos, Juan Ramón Oreja Rodríguez

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt73g.htm

Website: www.rasch.org/rmt/contents.htm