The Efficacy of Warm's Weighted Mean Likelihood Estimate (WLE) Correction to Maximum Likelihood Estimate (MLE) Bias

Thomas Warm (1989) reports that "Lord (1983) found that maximum likelihood estimates of θ [person ability] are biased outward" and then he restates Lord's expression for the size of this bias:

Bias (θ_MLE) = - J / ( 2 * I² )

where, for dichotomous Rasch items,
J = Σ P_θi (1-P_θi ) (1-2P_θi )
I = Σ P_θi (1-P_θi )
summed for all items, i = 1,L in the test, where P_θi is the Rasch-model probability of success of ability θ on item i.

The corrected estimate, θ_WLE = θ_MLE + (J / ( 2 * I² ) ), which is almost always closer to the item mean than θ_MLE.

How effective is this bias correction? Warm uses a Monte Carlo study to demonstrate its effectiveness, but an exact algebraic investigation can be conducted.

Dichotomous Items

I posited a test of 25 items, with its item difficulties uniformly spaced 0.2 logits apart. Figure 1 shows the locations (x-axis) of the items on the 25-item test. The item difficulties are centered on 0 logits.

Applying the MLE method of Wright & Douglas (1996) for estimating θ from known item difficulties, a Rasch ability estimate, M(s) is obtained for each possible raw score, s=0-25, on the test of 25 items. Since the estimates corresponding to s=0 and s=25 are infinite, they are substituted by estimates corresponding to s=0.3 and s=24.7 score-points. The MLE ability estimates are shown in Figure 1.

Figure 1. MLE and WLE for 25 dichotomous items.

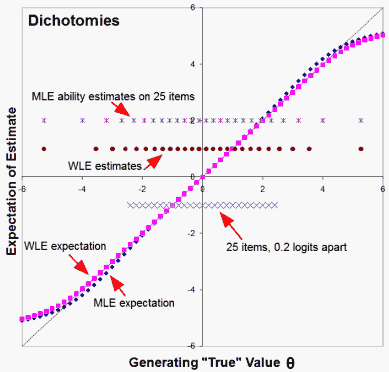
Warm's bias correction is applied to each MLE estimate, M(s), to produce a Warm's Mean Likelihood Estimation (WLE) value, W(s). See Figure 1. WLE estimates are more central than the MLE estimates, except for estimates corresponding to scores of 0.3 and 24.7, where the MLE estimates are used unchanged.

Under Rasch model conditions, each raw score, s, on a given set of items, corresponds to one estimated ability θ(s), but each true (generating) ability corresponds to all possible raw scores. For 25 items, there are 2^25 = 33,554,432 possible different response strings. According to the Rasch model, each of these response strings has a finite probability of being observed for each generating ability.

Probability of response string n for ability θ = Pnθ = Π exp( (xni (θ - di) ) / (1 + exp(θ - di) )

for i = 1 to 25, where xni is the scored 0,1 response to item i in response string n, and di is the difficulty of item i.

Response string n has a raw score of s = Σ xni for i = 1 to 25. Score s has an MLE estimate of Mn = M(s) and a WLE estimate of Wn = W(s).

The expected values of the estimates corresponding to each generating value can now be computed:

Expectation (MLE(θ)) = Σ Pnθ Mn for n = 1 to 2^25

Expectation (WLE(θ)) = Σ Pnθ Wn for n = 1 to 2^25

These values are plotted in Figure 1 for θ in the range -6 logits to +6 logits. The WLE ogive corresponds to the identity line with the generating values for most of its range. The MLE ogive is slightly less central (as predicted by Fred Lord). We can see that the WLE bias correction is effective over the entire range of MLE estimates for non-extreme scores (-4 to +4 logits). The biggest bias correction is 0.23 logits at a generating value of 3.6 logits, as shown in Figure 2. This is less than half the size of the standard error of each estimate which is close to 0.5 logits for most of the range. We can also see that, for "true" generating abilities within 2 logits of the center of the test, the MLE bias is less than 0.1 logits, and so negligible for practical purposes.

Figure 2. Detail of Figure 1 showing MLE bias.

Similar investigations for tests of length 2 to 24 items demonstrated that the WLE bias correction is effective for tests of 7 dichotomous items or more.

Polytomous Items

We can apply the same logic to Rasch-model polytomous items.

Bias (θ_MLE) = - J / ( 2 * I² )

J = = Σ Σ (P'_θikP"_θik)/P_θik = Σ( (Σk³P_θik ) - 3(Σk²P_θik )(ΣkP_θik ) + 2(ΣkP_θik )³ )

I = Σ( (Σk²P_θik ) - (ΣkP_θik )² )

where P_θik is the Rasch-model probability of a person of ability θ being observed in category k of item i, and the summations are over i=1, L and the polytomous categories k=0,m.

The corrected estimate, θ_WLE = θ_MLE + (J / ( 2 * I² ) ), which is almost always closer to the item mean than θ_MLE.

The results of this investigation are shown in Figure 3 with items 0.1 logits apart, and thresholds 1 logit apart. Using the estimation procedure in Linacre (1998), the results are similar to the findings for dichotomous items in Figure 1.

Warm's bias correction is seen to be efficacious for the correction of MLE bias across the useful measurement range of the items, but MLE bias is also seen to be inconsequential for most practical purposes.

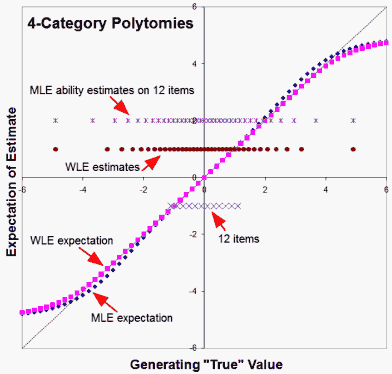
Figure 3. MLE and WLE for 12, 4-category, items.

John M. Linacre

Linacre J.M. (1998) Estimating Rasch measures with known polytomous (or rating scale) item difficulties: Anchored Maximum Likelihood Estimation (AMLE), RMT 12:2 p. 638

Lord, F. M. (1983). Unbiased estimators of ability parameters, of their variance, and of their parallel-forms reliability. Psychometrika, 48, 2, 233-245.

Warm T.A. (1989) Weighted Likelihood Estimation of Ability in Item Response Theory. Psychometrika, 54, 427-450.

Wright B.D., Douglas G.A. (1996) Estimating measures with known dichotomous item difficulties. Rasch Measurement Transactions, 10:2, 499

---

Linacre J.M. (2009) The Efficacy of Warm's Weighted Mean Likelihood Estimate (WLE) Correction to Maximum Likelihood Estimate (MLE) Bias, Rasch Measurement Transactions, 2009, 23:1, 1188-9

Rasch Books and Publications
Invariant Measurement: Using Rasch Models in the Social, Behavioral, and Health Sciences, 2nd Edn. George Engelhard, Jr. & Jue Wang	Applying the Rasch Model (Winsteps, Facets) 4th Ed., Bond, Yan, Heene	Advances in Rasch Analyses in the Human Sciences (Winsteps, Facets) 1st Ed., Boone, Staver	Advances in Applications of Rasch Measurement in Science Education, X. Liu & W. J. Boone	Rasch Analysis in the Human Sciences (Winsteps) Boone, Staver, Yale
Introduction to Many-Facet Rasch Measurement (Facets), Thomas Eckes	Statistical Analyses for Language Testers (Facets), Rita Green	Invariant Measurement with Raters and Rating Scales: Rasch Models for Rater-Mediated Assessments (Facets), George Engelhard, Jr. & Stefanie Wind	Aplicação do Modelo de Rasch (Português), de Bond, Trevor G., Fox, Christine M	Appliquer le modèle de Rasch: Défis et pistes de solution (Winsteps) E. Dionne, S. Béland
Exploring Rating Scale Functioning for Survey Research (R, Facets), Stefanie Wind	Rasch Measurement: Applications, Khine	Winsteps Tutorials - free Facets Tutorials - free	Many-Facet Rasch Measurement (Facets) - free, J.M. Linacre	Fairness, Justice and Language Assessment (Winsteps, Facets), McNamara, Knoch, Fan
Other Rasch-Related Resources: Rasch Measurement YouTube Channel
Rasch Measurement Transactions & Rasch Measurement research papers - free	An Introduction to the Rasch Model with Examples in R (eRm, etc.), Debelak, Strobl, Zeigenfuse	Rasch Measurement Theory Analysis in R, Wind, Hua	Applying the Rasch Model in Social Sciences Using R, Lamprianou	El modelo métrico de Rasch: Fundamentación, implementación e interpretación de la medida en ciencias sociales (Spanish Edition), Manuel González-Montesinos M.
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Rasch Models for Measurement, David Andrich	Constructing Measures, Mark Wilson	Best Test Design - free, Wright & Stone Rating Scale Analysis - free, Wright & Masters
Virtual Standard Setting: Setting Cut Scores, Charalambos Kollias	Diseño de Mejores Pruebas - free, Spanish Best Test Design	A Course in Rasch Measurement Theory, Andrich, Marais	Rasch Models in Health, Christensen, Kreiner, Mesba	Multivariate and Mixture Distribution Rasch Models, von Davier, Carstensen

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt231d.htm

Website: www.rasch.org/rmt/contents.htm