Item Discrimination Indices

Item discrimination indicates the extent to which success on an item corresponds to success on the whole test. Since all items in a test are intended to cooperate to generate an overall test score, any item with negative or zero discrimination undermines the test. Positive item discrimination is generally productive, unless it is so high that the item merely repeats the information provided by other items on the test. This is the "attenuation paradox."

The Discrimination Index (D) is computed from equal-sized high and low scoring groups on the test. Subtract the number of successes by the low group on the item from the number of successes by the high group, and divide this difference by the size of a group. The range of this index is +1 to -1. Using Truman Kelley's "27% of sample" group size, values of 0.4 and above are regarded as high and less than 0.2 as low by R.L. Ebel (1954, Procedures for the Analysis of Classroom Tests, Educational and Psychological Measurement, 14, 352-364).

The Point-biserial Correlation is the Pearson correlation between responses to a particular item and scores on the total test (with or without that item). The Biserial Correlation models the responses to the item to represent stratification of a normal distribution and computes the correlation accordingly. Again the ranges are +1 to -1. The biserial is always more extreme than the point-biserial. Jm Nunnally (Psychometric Theory, 1967, p. 123) states that "to use the biserial is to paint a faulty picture of the actual size of the correlations obtainable from existing data." A convenient substitute for these correlations, particularly when data are missing, is the correlation between the Rasch person measures and their responses to the item, the point-measure correlation.

The 2-PL model parameterizes item discrimination in the model and uses it to estimate person ability. A 2-PL model can be written:

(1)

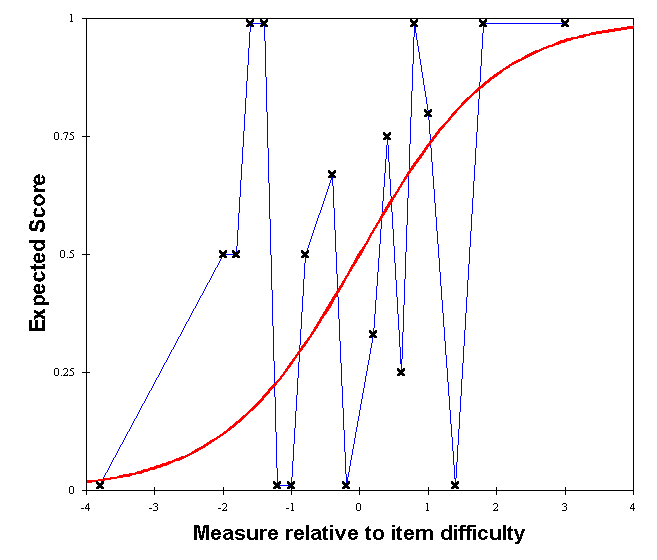
At its core, the estimation process is:

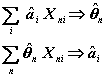
(2)   (3)

Unconstrained, this produces a feed-back loop. In (2), success on highly discriminating items (X_ni=1) raises the person measure, failure (X_ni=0) lowers the person measure. In (3), success on an item by those with high measures, coupled with failure by those with low measures, raises the item discrimination. This raised discrimination then feeds back into (2) to increase the measure difference between the successful and unsuccessful, which then, in (3), increases the item discrimination, ad infinitum. To avoid this, 2-PL estimation programs introduce constraints such as a maximum limit on item discrimination estimates, and a pre-set person distribution. Rasch models have pre-set item discriminations in the model, so feedback does not occur. Person and item measures can be estimated in (2) because a_i is set to 1 at this step. Then those measures can be used in (3) to estimate Rasch" item discriminations. There is no return to (2).

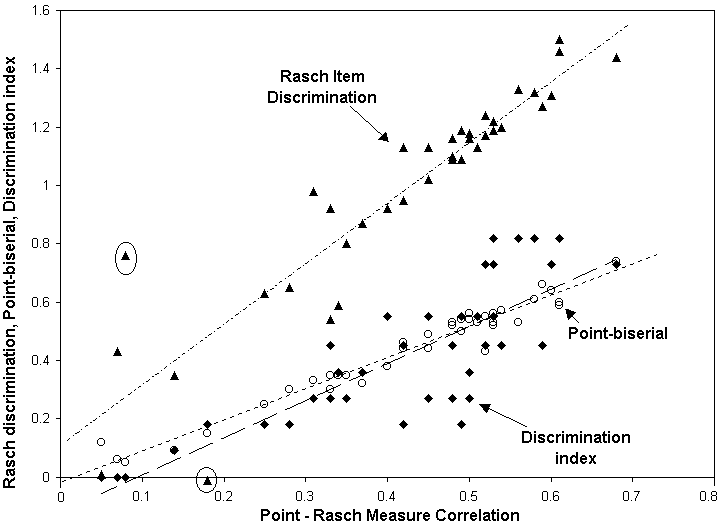
The plot shows the relationship between these indices. It reports item discrimination indices for dichotomous data reported in W J. Micheels and M. R. Karnes (1950, Measuring Educational Achievement, p. 478-9). For these data, the biserial correlations sometimes exceed 1, so that index is contra-indicated. The Discrimination Index (D) has been computed with the top 27% of the person sample in the high group and the bottom 27% in the low group. The trendlines (.... point-biserial, - - - discrimination index) show that all indices give similar information. The item ringed on left side of the plot has a low correlation but high Rasch item discrimination. It is an easy item with a few misfitting incorrect responses. The item ringed in the bottom left of the plot has negative Rasch discrimination. Its model and empirical ICCs are shown here. These results suggest that the point-measure or, for complete data, the point-biserial correlation capture the useful item discrimination information.

John M. Linacre

Note: the biserial correlation originated in Karl Pearson, "On a New Method of Determining Correlation ....", Biometrika, Vol. VII, pp. 96-105, 1909, and the point-biserial correlation originated in Richardson, M.W. & Stalnaker, J.M. (1933). "A note on the use of bi-serial r in test research". Journal of General Psychology, 8, 463-465.

Item 31 (Micheels & Karnes)

1. The Item Discrimination Index: Does it Work? Tristan Lopez A. … Rasch Measurement Transactions, 1998, 12:1 p. 626
2. BICAL item discrimination index. Wright BD, Mead RJ, Bell SR. … Rasch Measurement Transactions, 2002, 16:1 p.869
3. Item Discrimination Indices. Kelley T., Ebel R., Linacre, JM. … Rasch Measurement Transactions, 2002, 16:3 p.883-4

4. Discrimination, Guessing and Carelessness Asymptotes: Estimating IRT Parameters with Rasch. Linacre J.M. … Rasch Measurement Transactions, 2004, 18:1 p.959-960

Item Discrimination Indices. Kelley T., Ebel R., Linacre, JM. … Rasch Measurement Transactions, 2002, 16:3 p.883-4

Rasch-Related Resources: Rasch Measurement YouTube Channel
Rasch Measurement Transactions & Rasch Measurement research papers - free	An Introduction to the Rasch Model with Examples in R (eRm, etc.), Debelak, Strobl, Zeigenfuse	Rasch Measurement Theory Analysis in R, Wind, Hua	Applying the Rasch Model in Social Sciences Using R, Lamprianou	El modelo métrico de Rasch: Fundamentación, implementación e interpretación de la medida en ciencias sociales (Spanish Edition), Manuel González-Montesinos M.
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Rasch Models for Measurement, David Andrich	Constructing Measures, Mark Wilson	Best Test Design - free, Wright & Stone Rating Scale Analysis - free, Wright & Masters
Virtual Standard Setting: Setting Cut Scores, Charalambos Kollias	Diseño de Mejores Pruebas - free, Spanish Best Test Design	A Course in Rasch Measurement Theory, Andrich, Marais	Rasch Models in Health, Christensen, Kreiner, Mesba	Multivariate and Mixture Distribution Rasch Models, von Davier, Carstensen
Rasch Books and Publications: Winsteps and Facets
Applying the Rasch Model (Winsteps, Facets) 4th Ed., Bond, Yan, Heene	Advances in Rasch Analyses in the Human Sciences (Winsteps, Facets) 1st Ed., Boone, Staver	Advances in Applications of Rasch Measurement in Science Education, X. Liu & W. J. Boone	Rasch Analysis in the Human Sciences (Winsteps) Boone, Staver, Yale	Appliquer le modèle de Rasch: Défis et pistes de solution (Winsteps) E. Dionne, S. Béland
Introduction to Many-Facet Rasch Measurement (Facets), Thomas Eckes	Rasch Models for Solving Measurement Problems (Facets), George Engelhard, Jr. & Jue Wang	Statistical Analyses for Language Testers (Facets), Rita Green	Invariant Measurement with Raters and Rating Scales: Rasch Models for Rater-Mediated Assessments (Facets), George Engelhard, Jr. & Stefanie Wind	Aplicação do Modelo de Rasch (Português), de Bond, Trevor G., Fox, Christine M
Exploring Rating Scale Functioning for Survey Research (R, Facets), Stefanie Wind	Rasch Measurement: Applications, Khine	Winsteps Tutorials - free Facets Tutorials - free	Many-Facet Rasch Measurement (Facets) - free, J.M. Linacre	Fairness, Justice and Language Assessment (Winsteps, Facets), McNamara, Knoch, Fan

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt163a.htm

Website: www.rasch.org/rmt/contents.htm